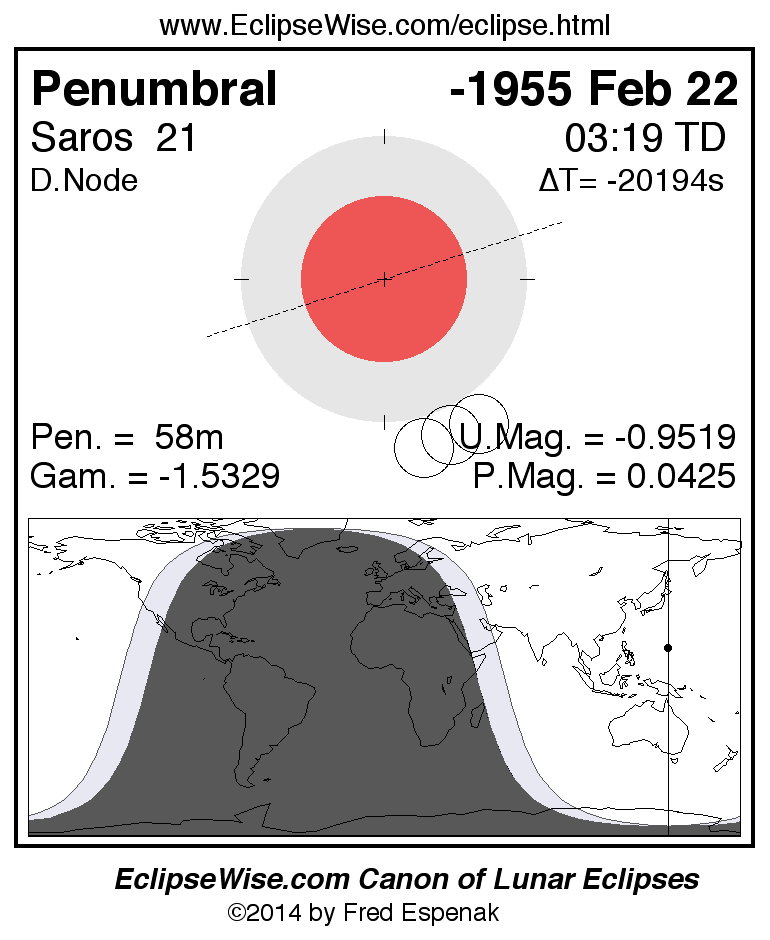
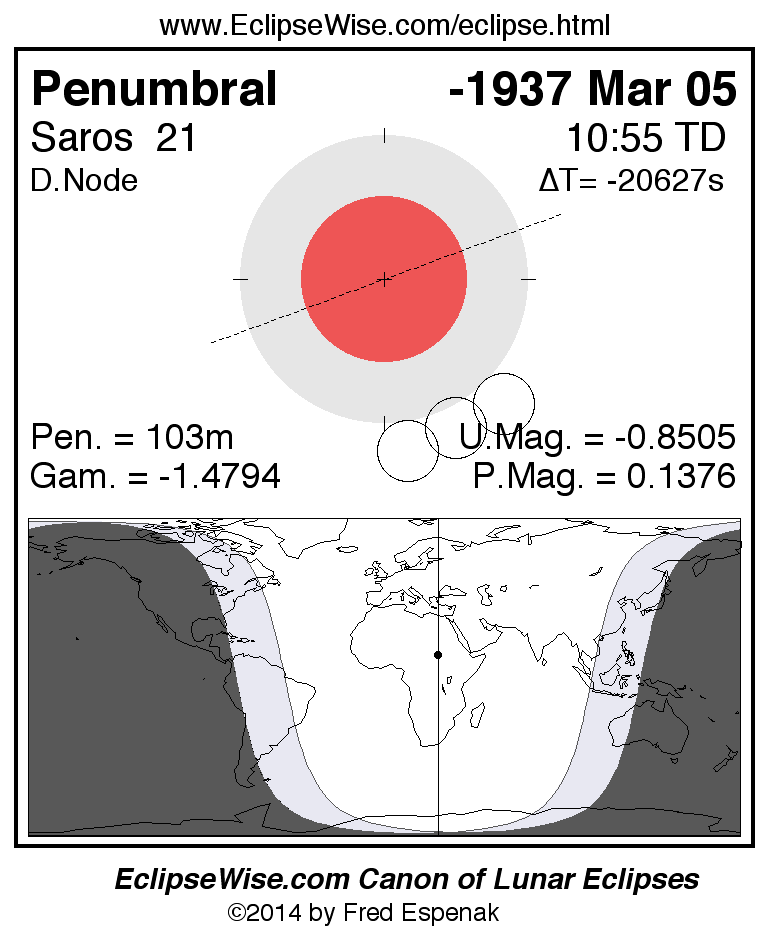
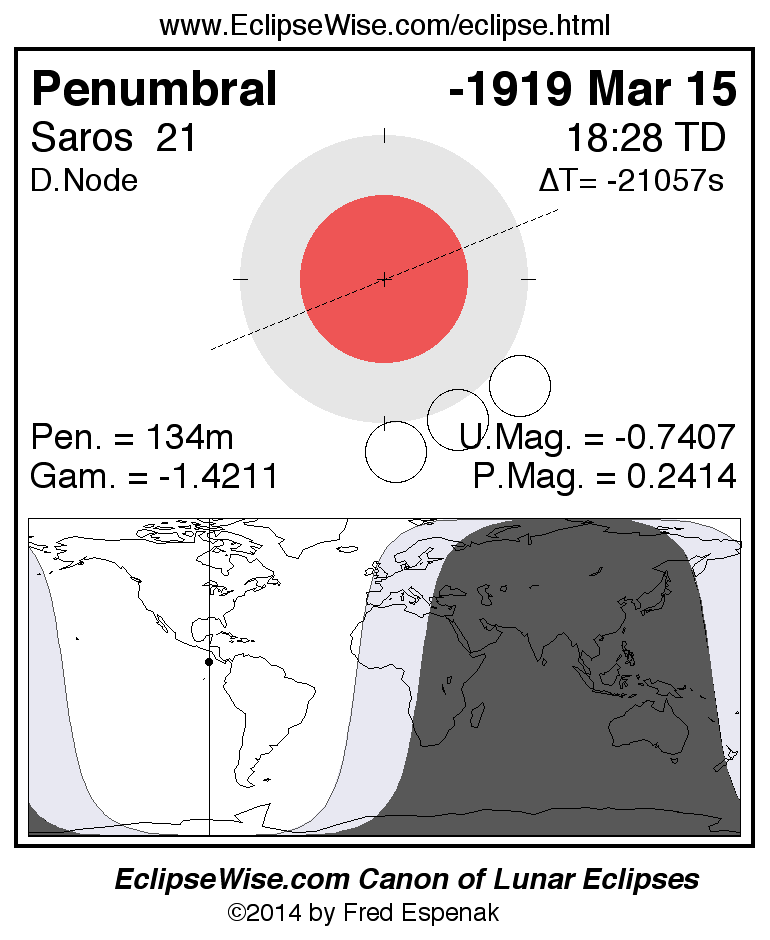
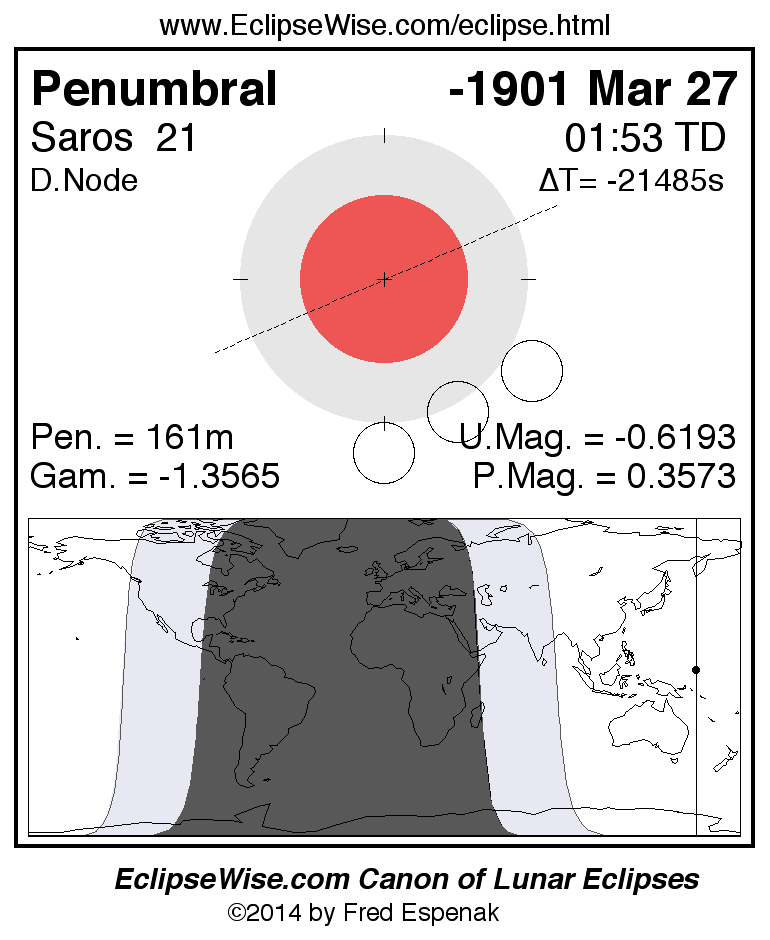
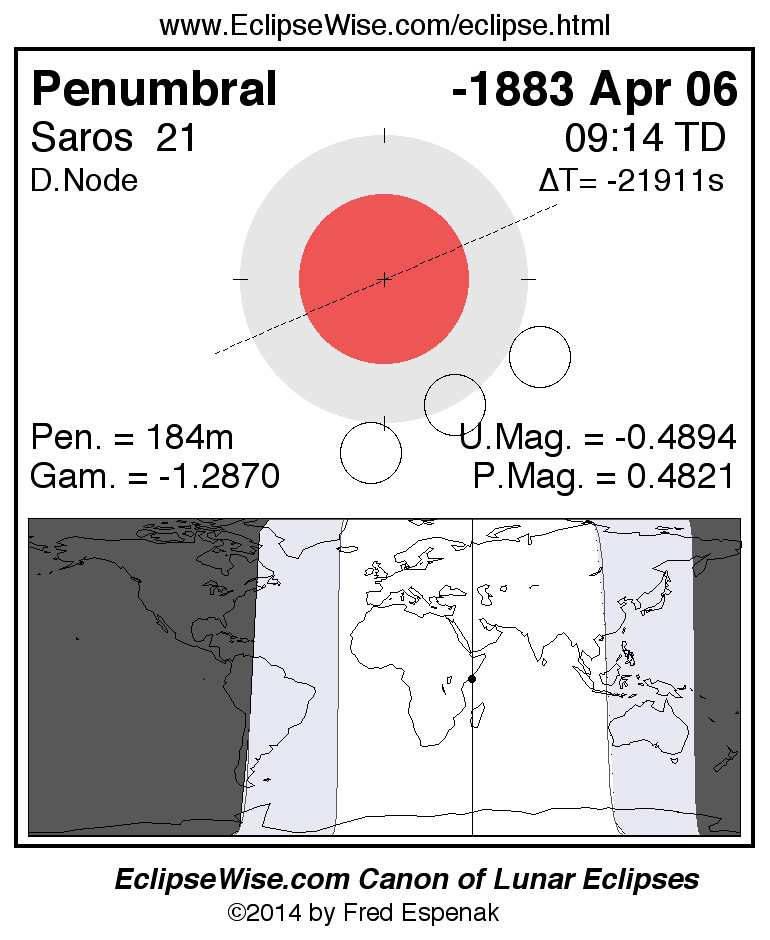
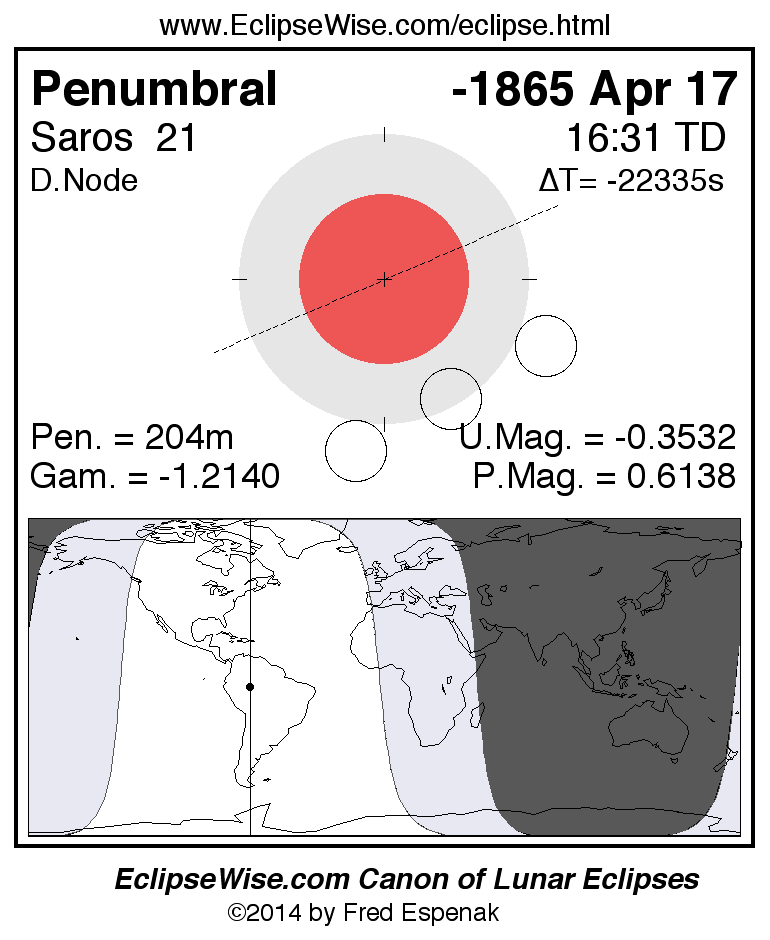
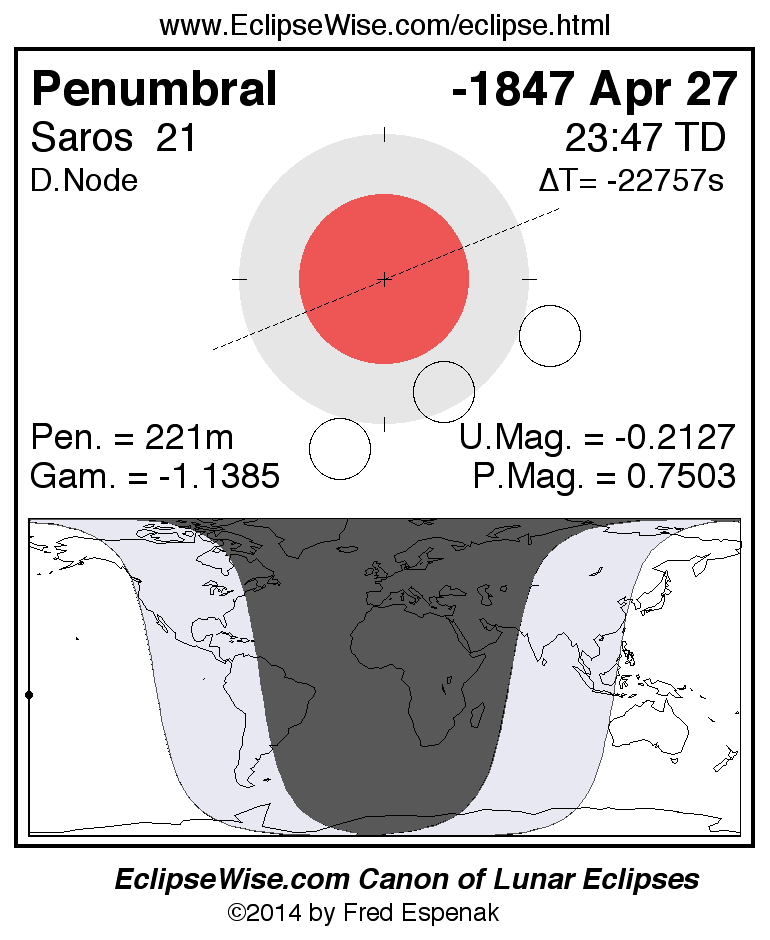
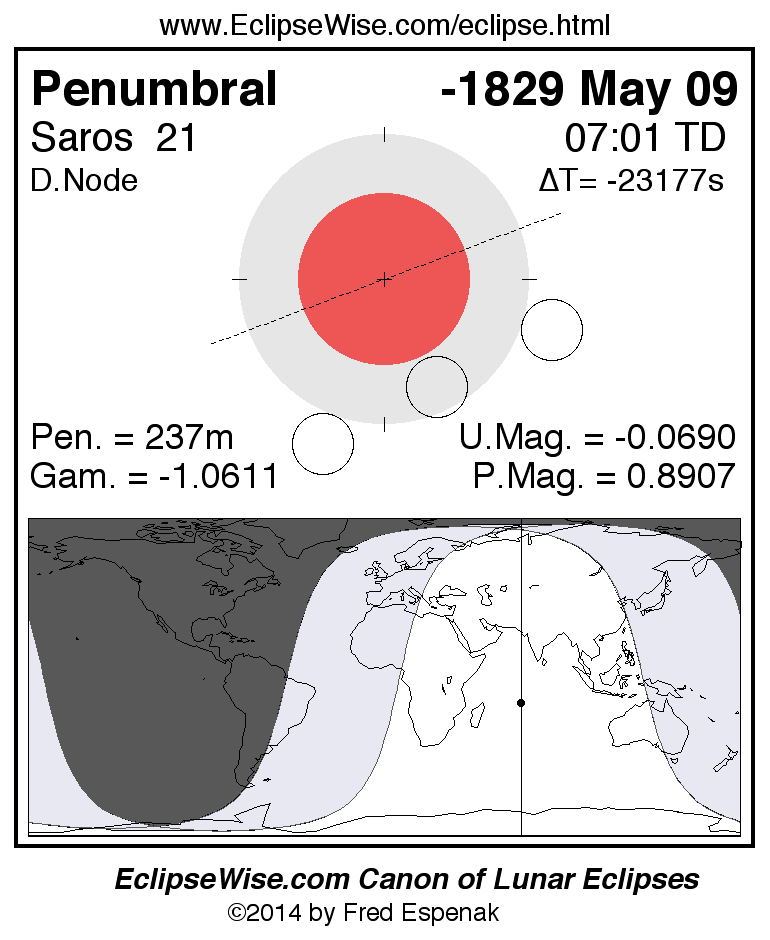
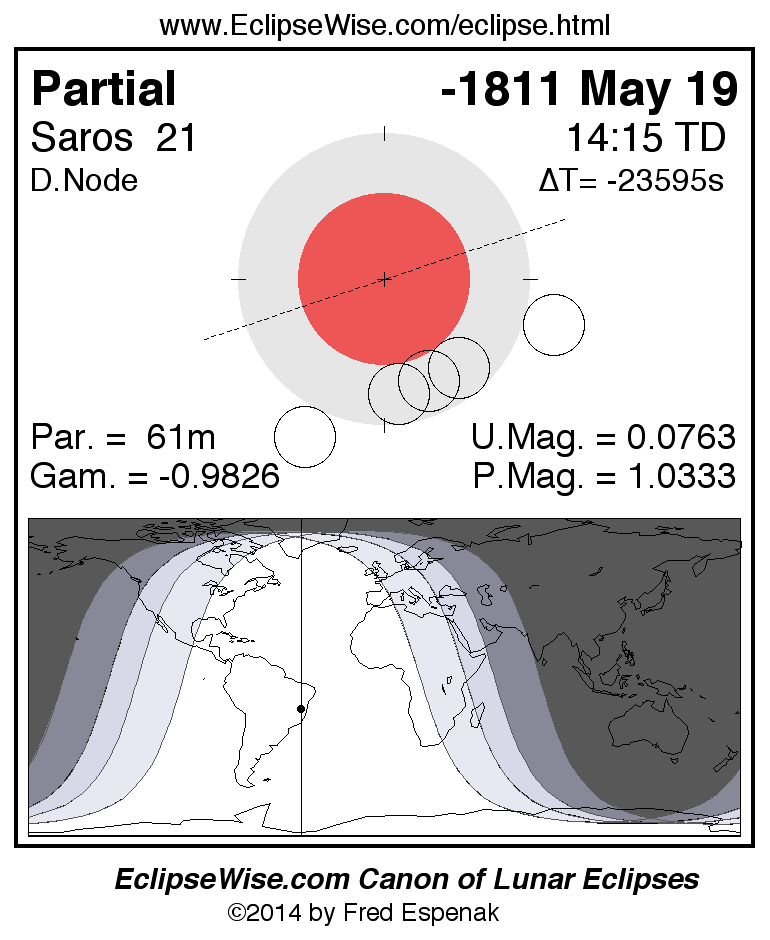
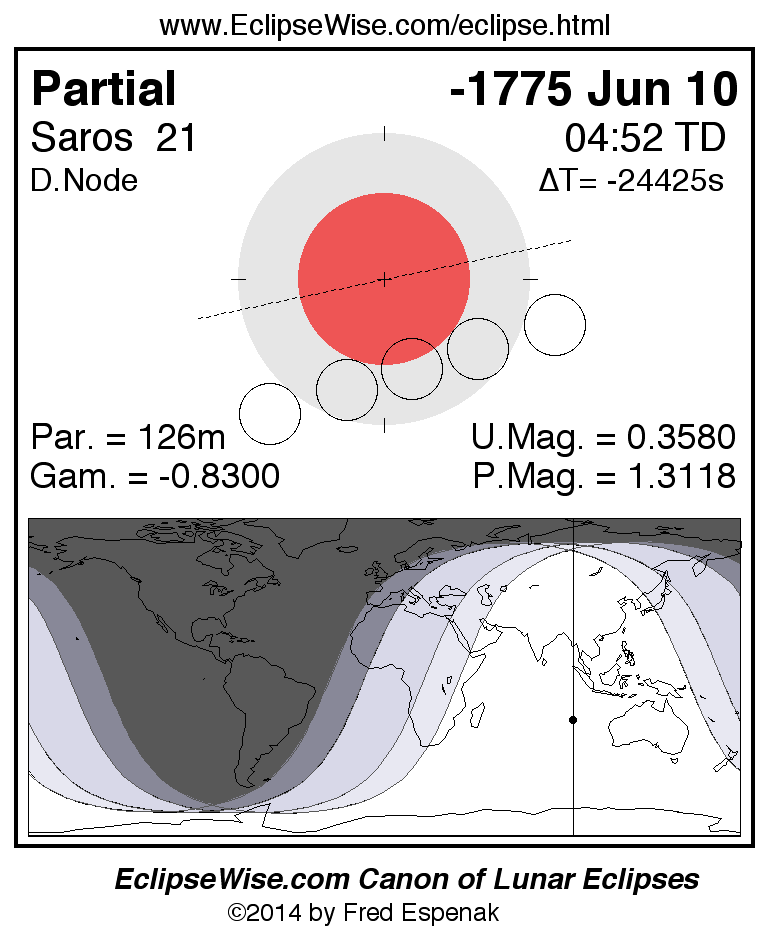
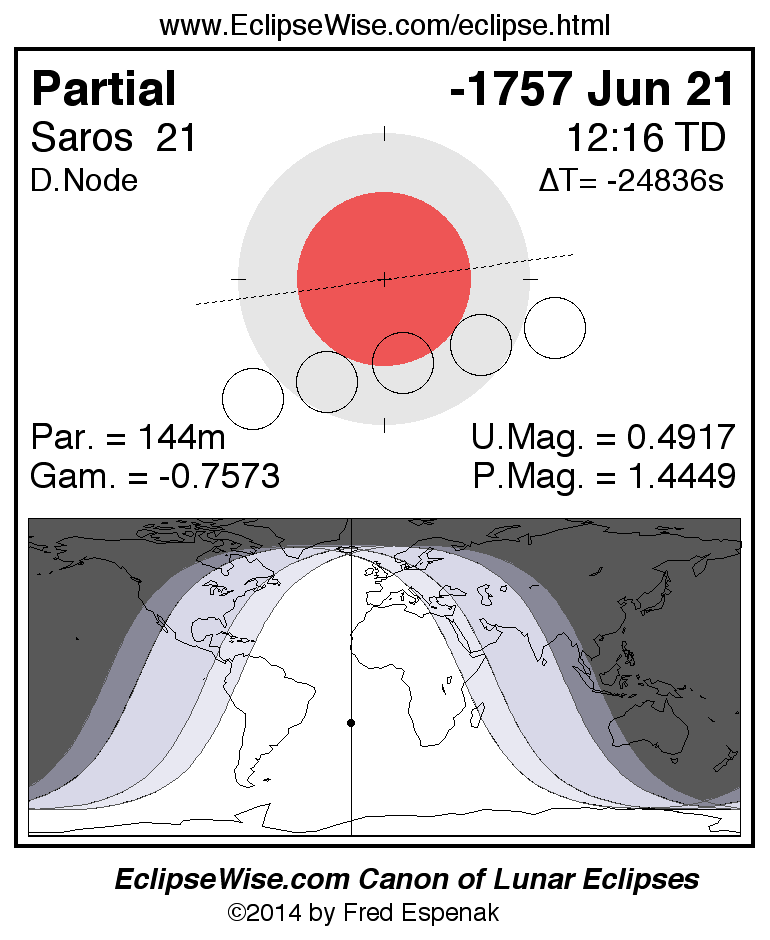
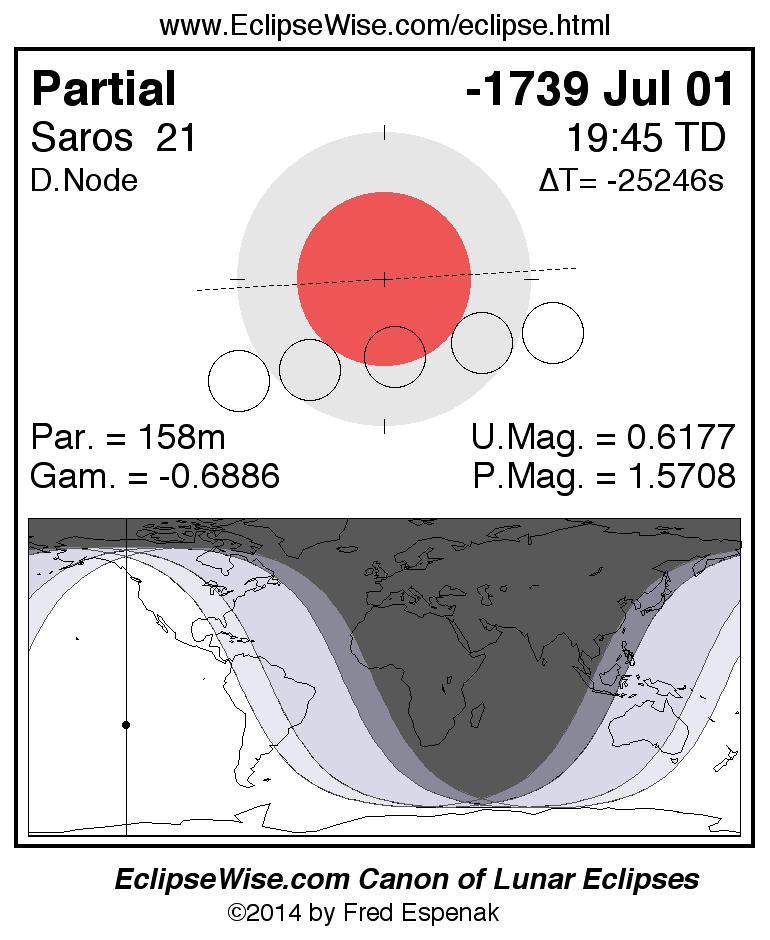
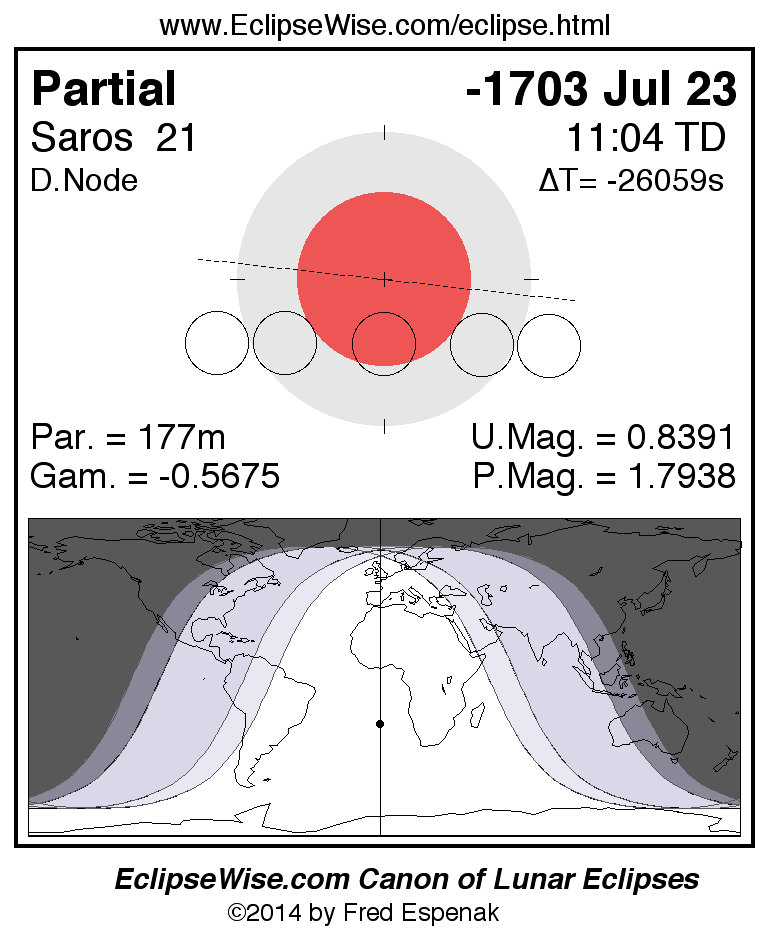
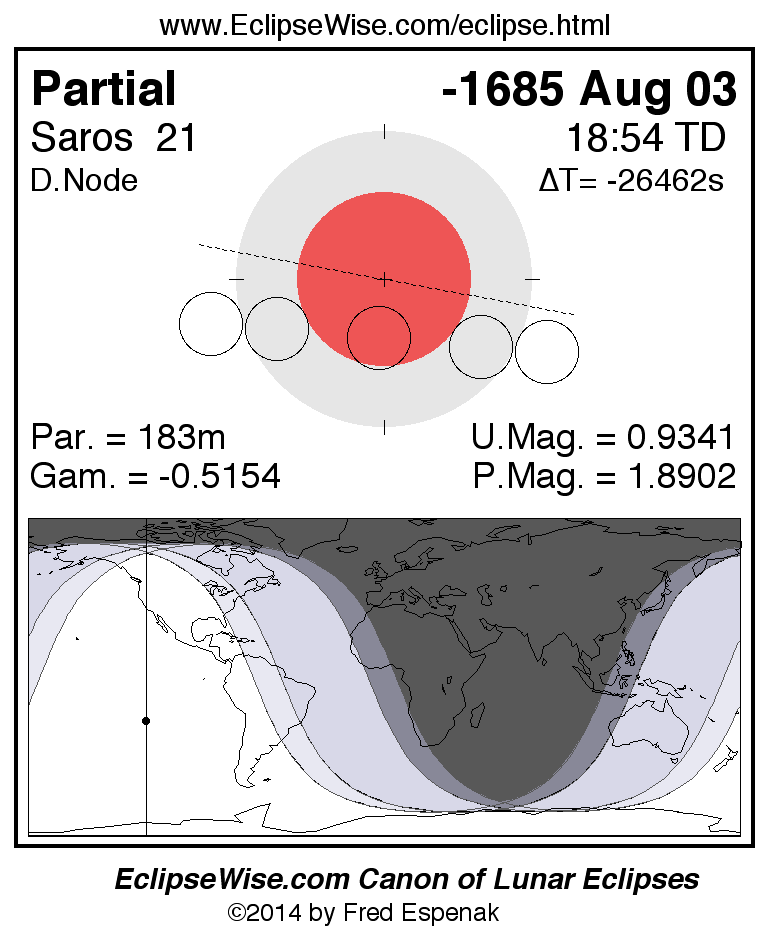
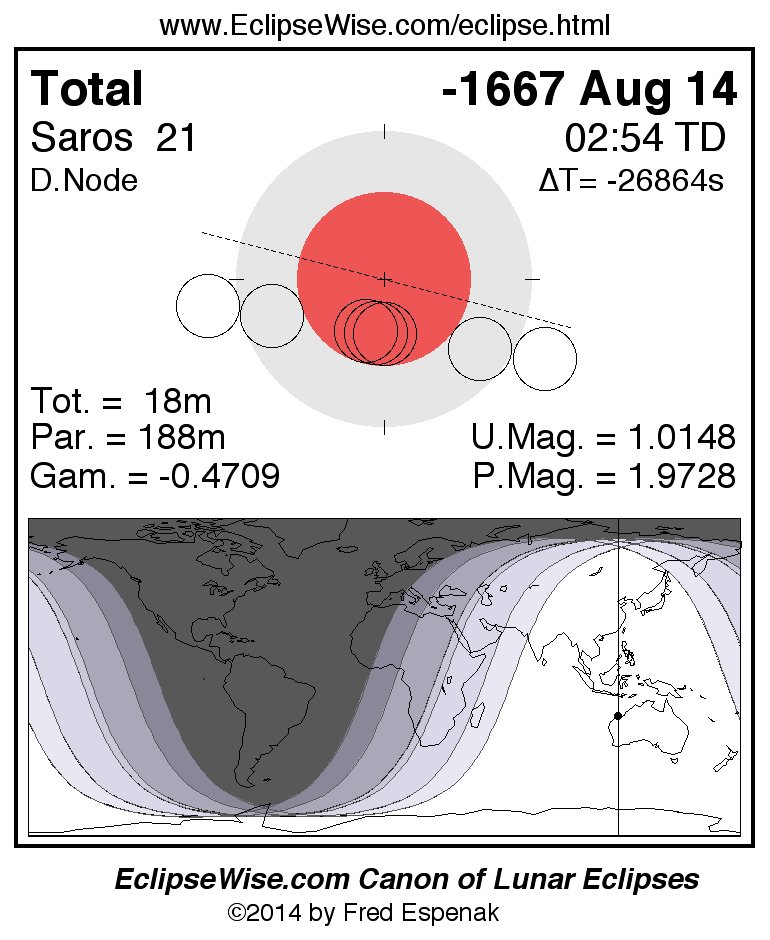
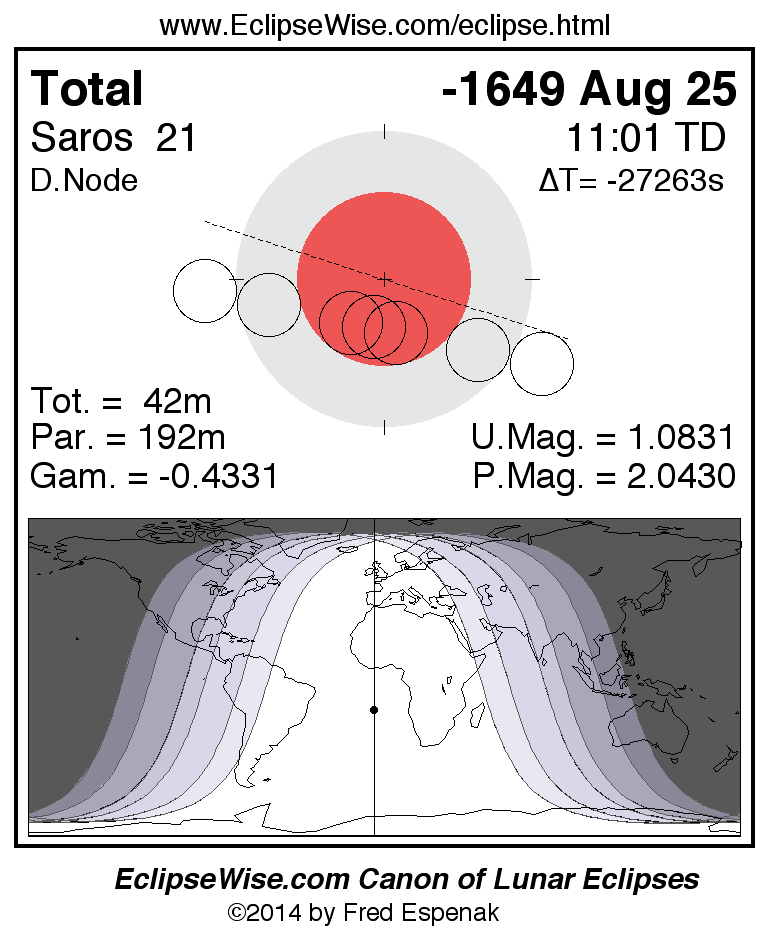
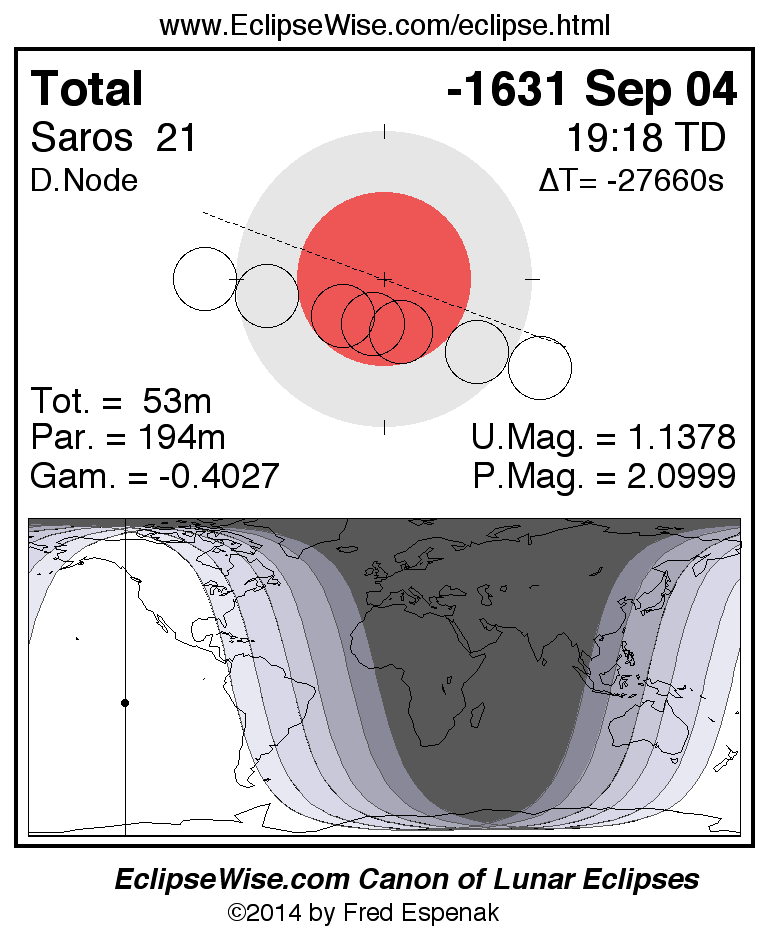
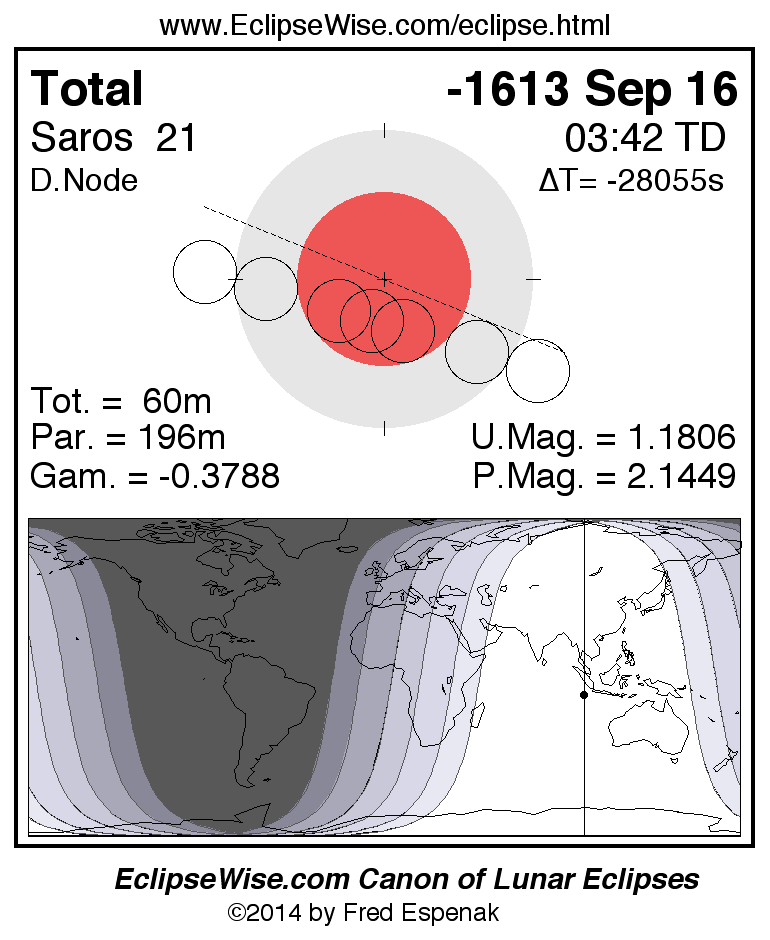
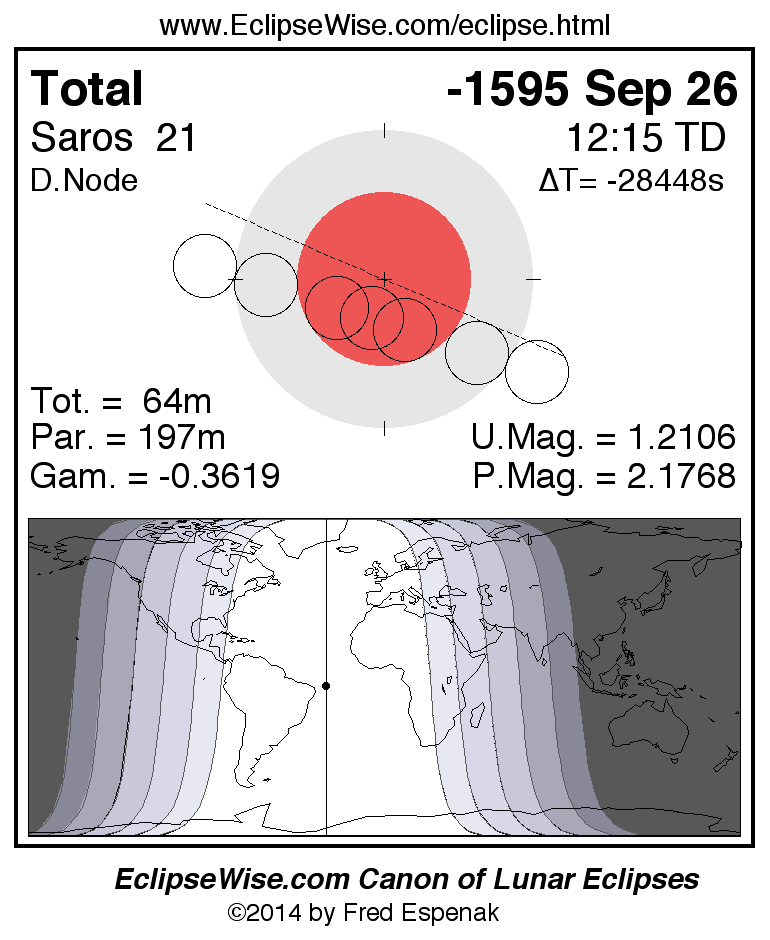
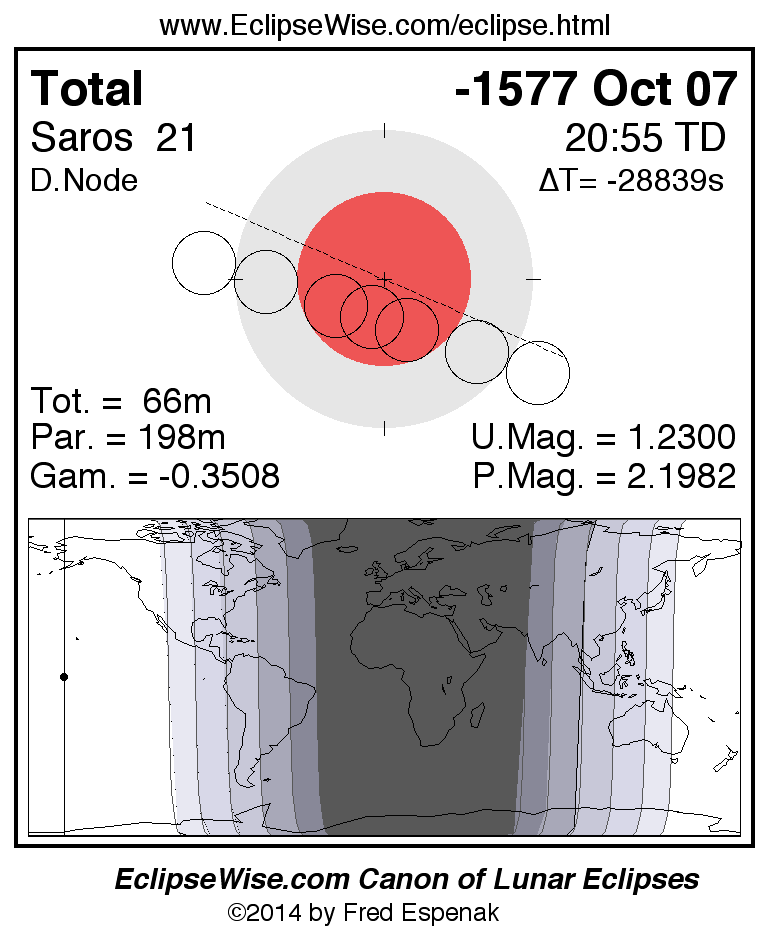
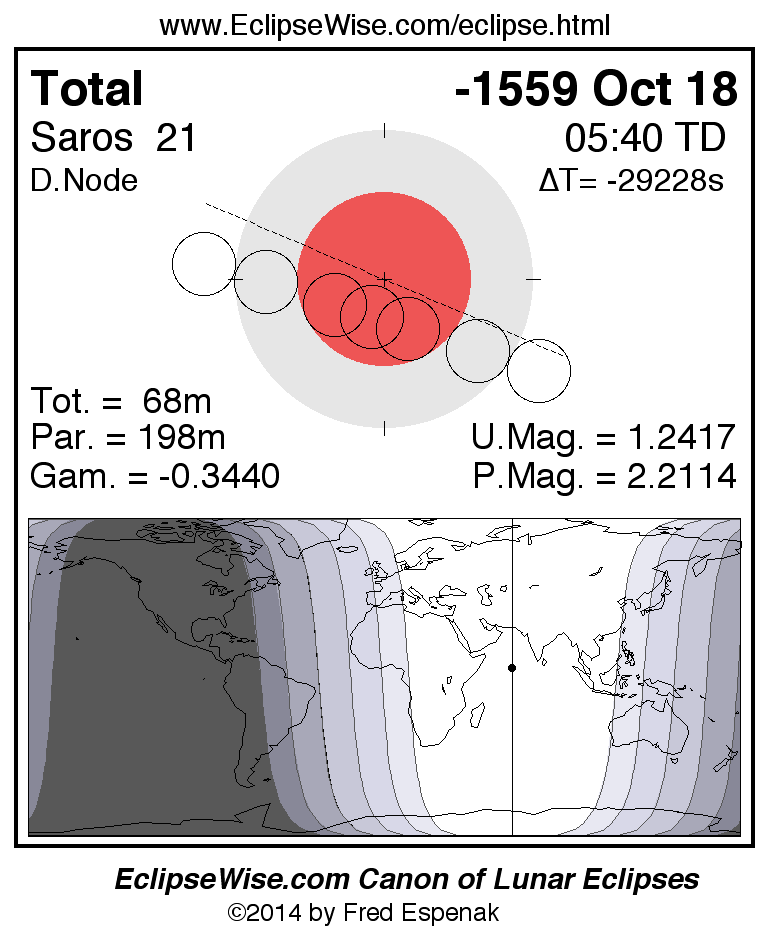
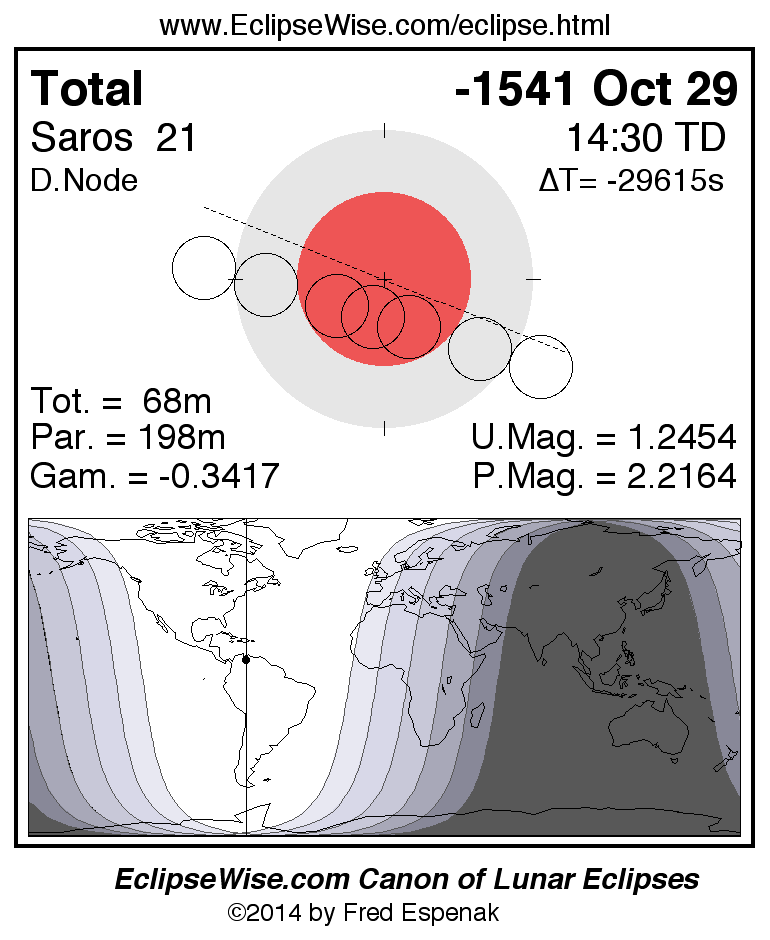
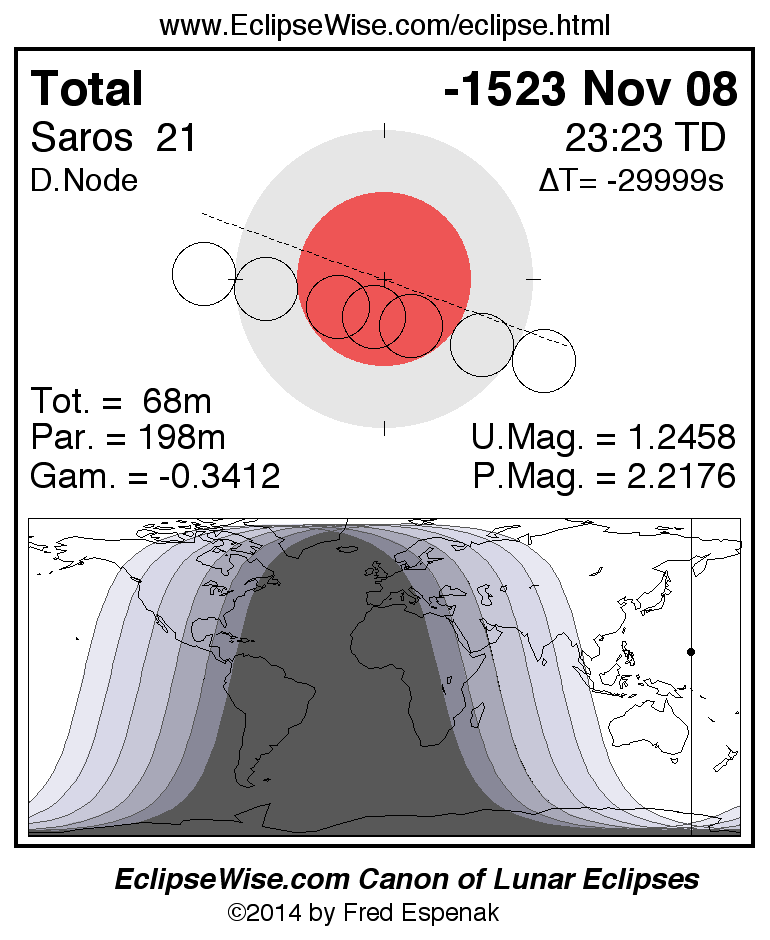
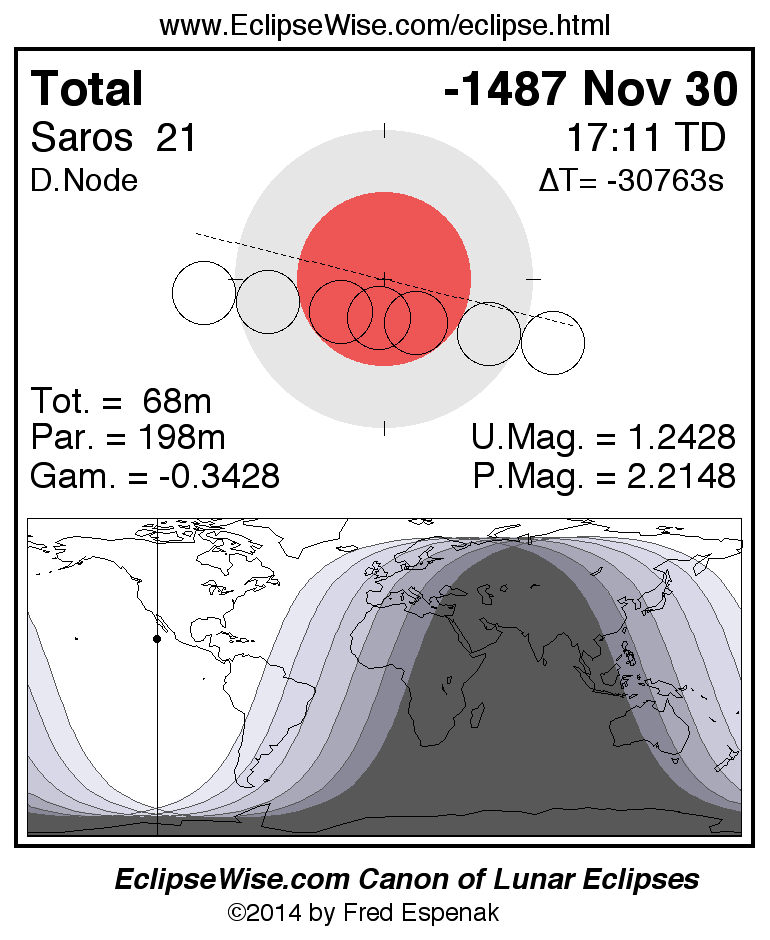
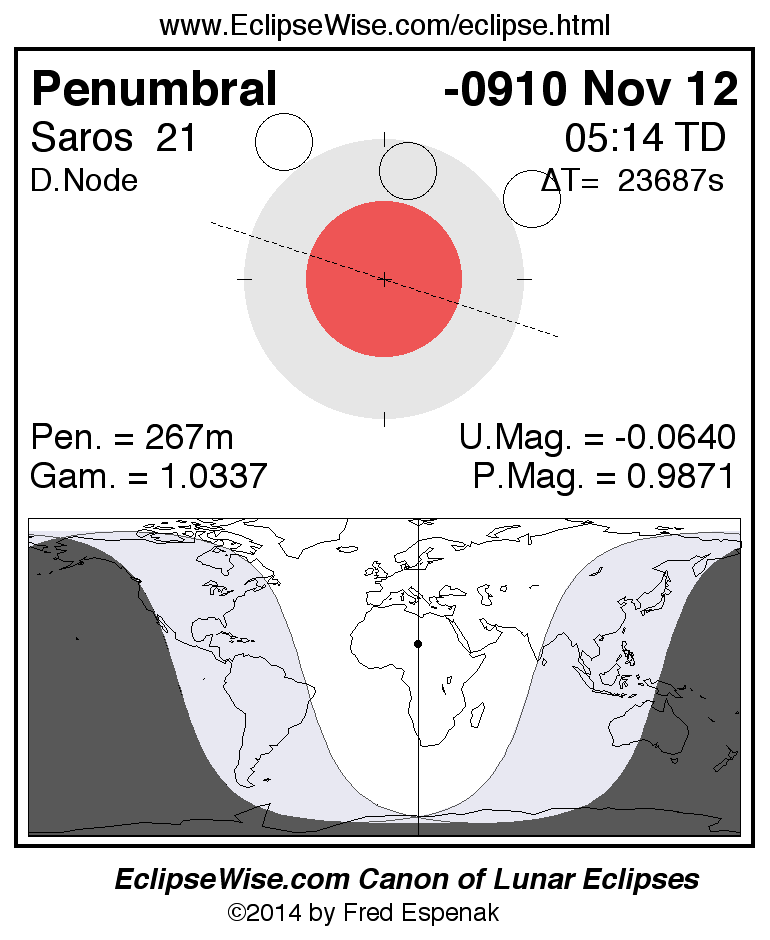
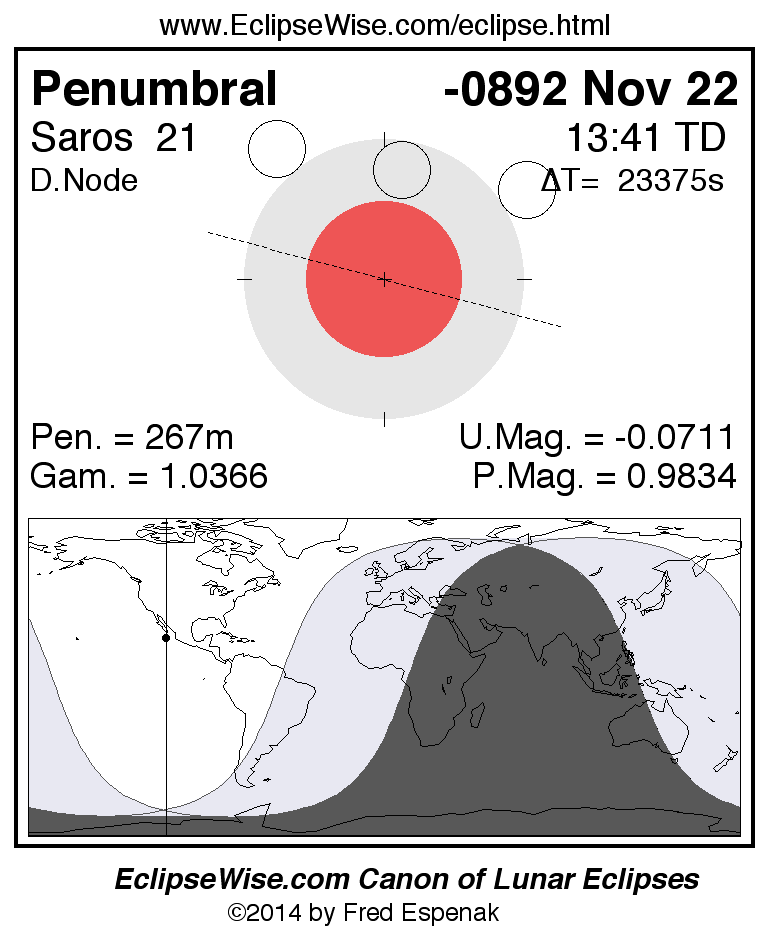
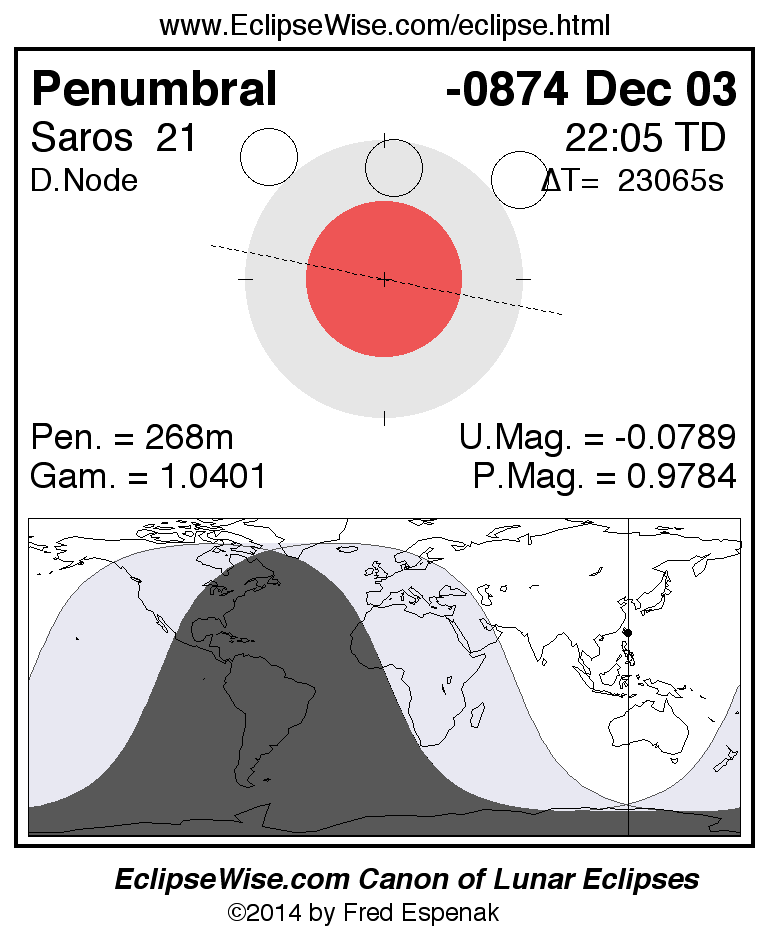
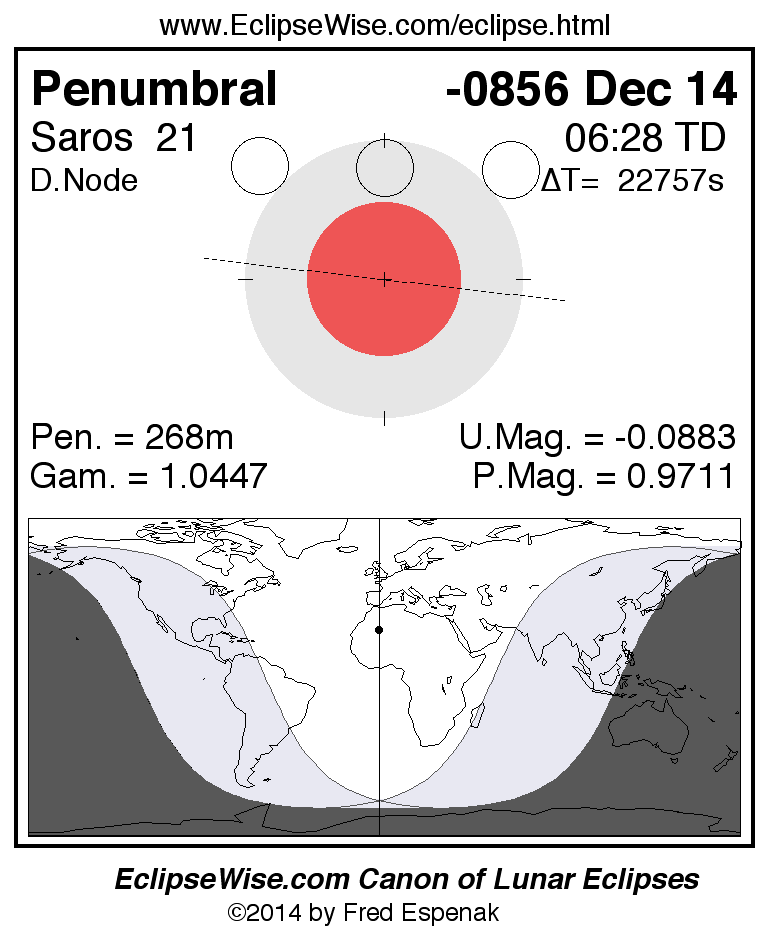
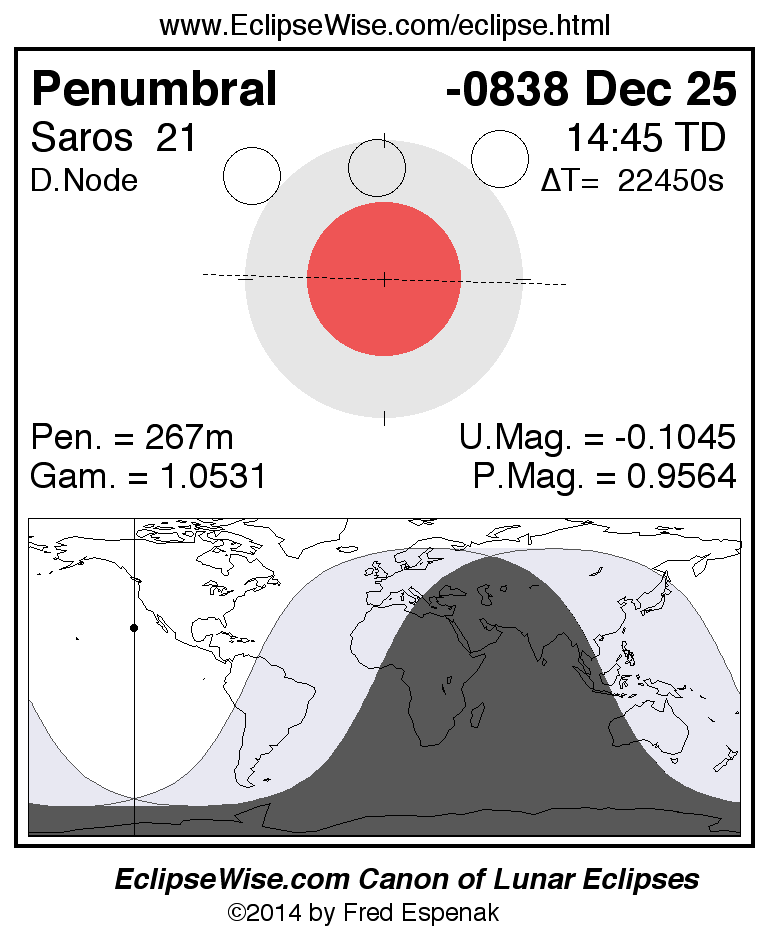
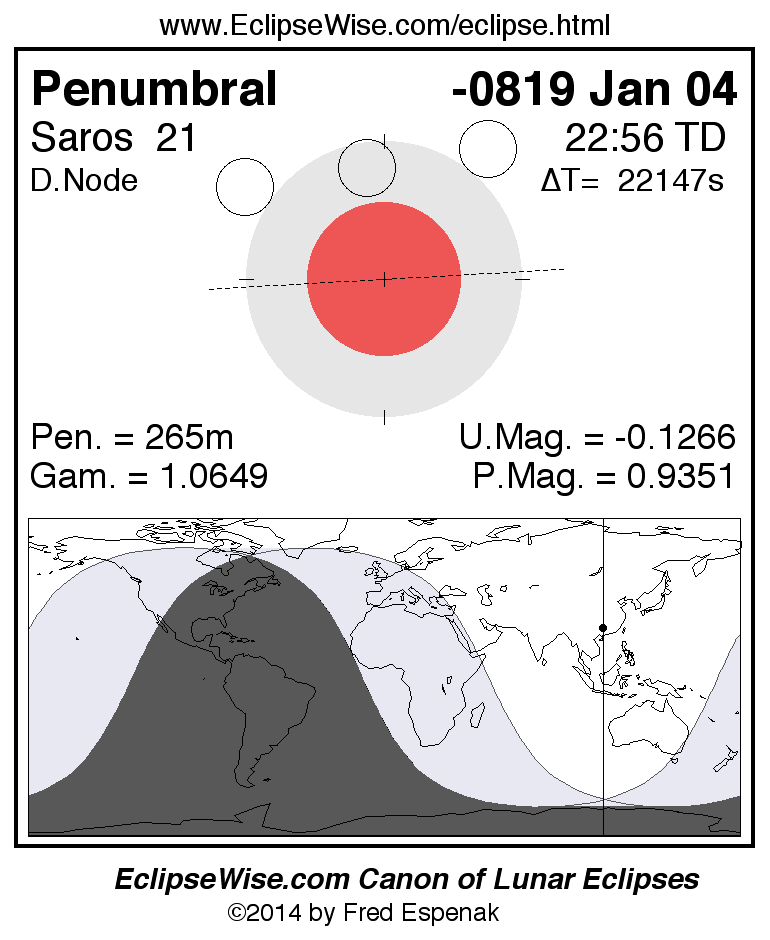
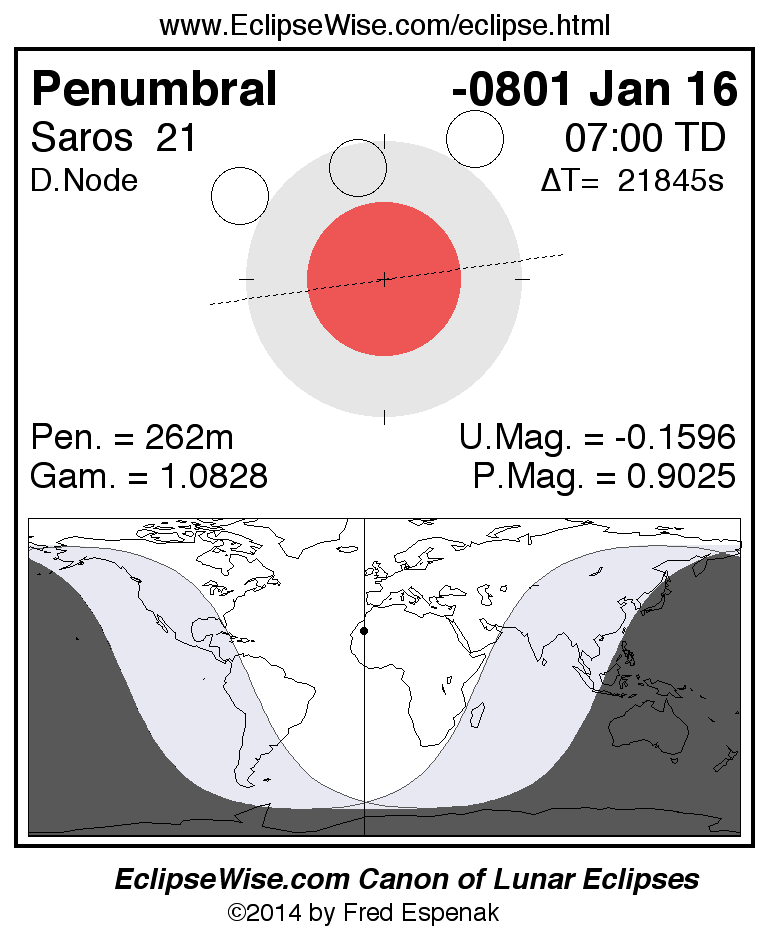
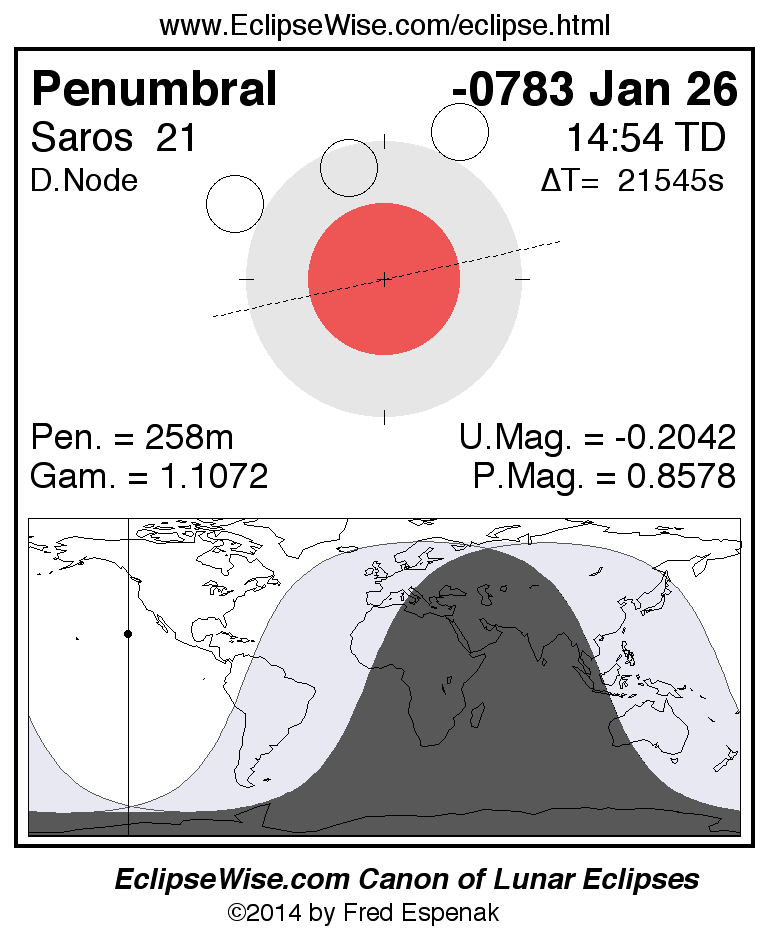
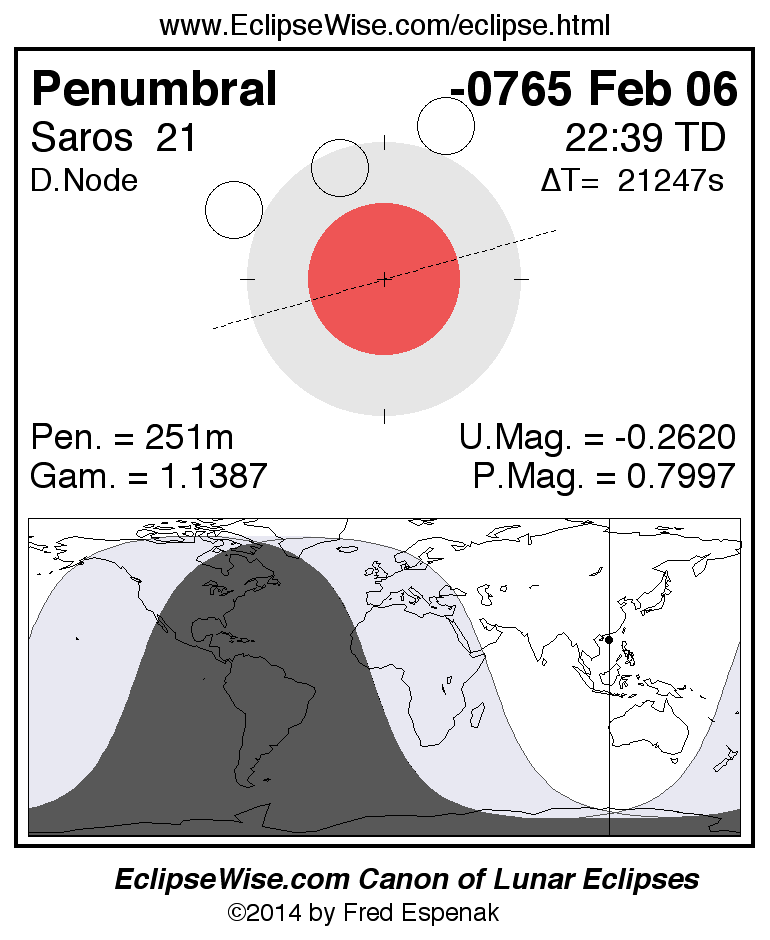
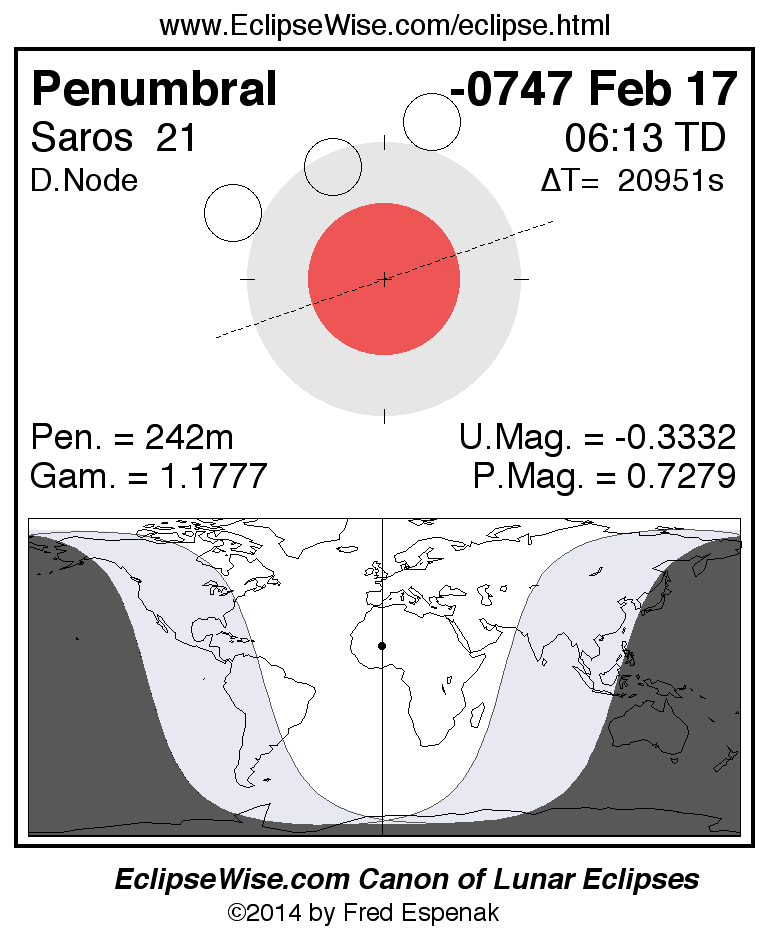
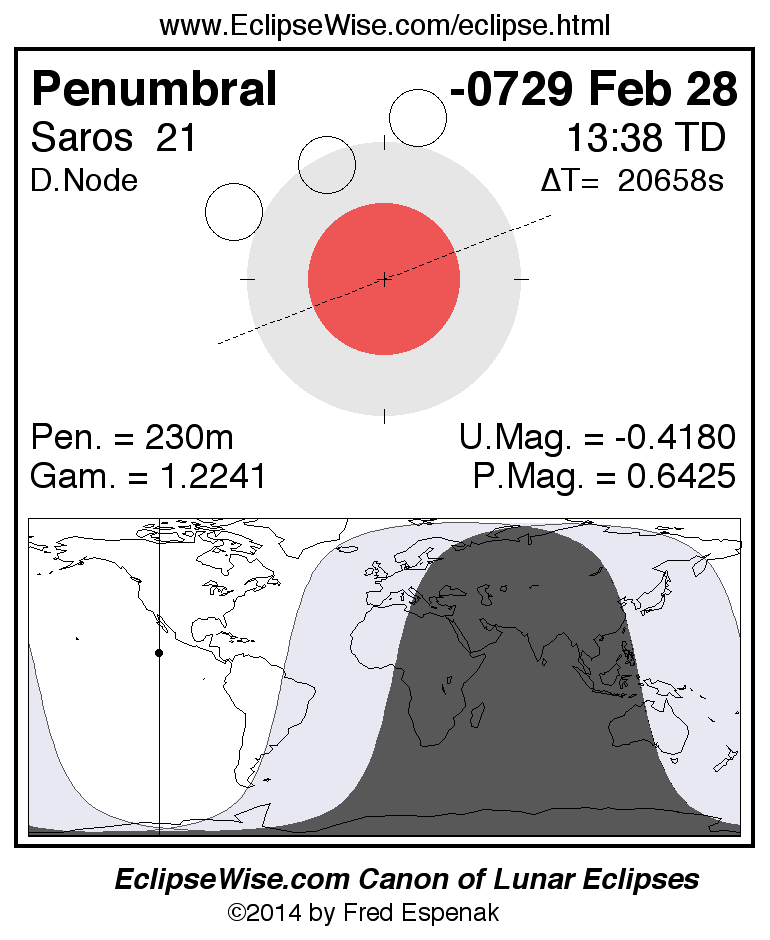
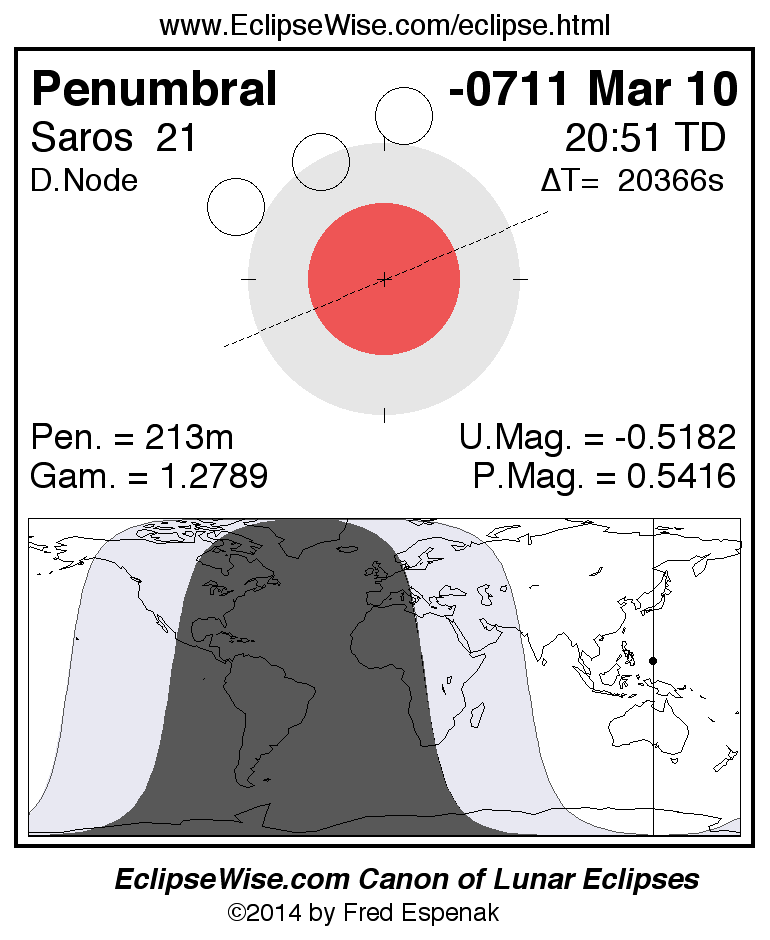
Saros 21
Panorama of Lunar Eclipses of Saros 21
Fred Espenak
Introduction
A lunar eclipse occurs whenever the Moon passes through Earth's shadow. At least two lunar eclipses and as many as five occur every year.
The periodicity and recurrence of lunar eclipses is governed by the Saros cycle, a period of approximately 6,585.3 days (18 years 11 days 8 hours). When two eclipses are separated by a period of one Saros, they share a very similar geometry. The two eclipses occur at the same node with the Moon at nearly the same distance from Earth and the same time of year due to a harmonic in three cycles of the Moon's orbit. Thus, the Saros is useful for organizing eclipses into families or series. Each series typically lasts 12 to 15 centuries and contains about 70 to 80 eclipses. Every saros series begins with a number of penumbral lunar eclipses. The series will then produce several dozen partial eclipses, followed by several dozen total eclipses. The later portion of the series produces another set of partial eclipses before ending with a final group of penumbral eclipses. The exact numbers vary from one series to the next, but the overall sequence remains the same. For more information, see Periodicity of Lunar Eclipses.
Panorama of Lunar Eclipses of Saros 21
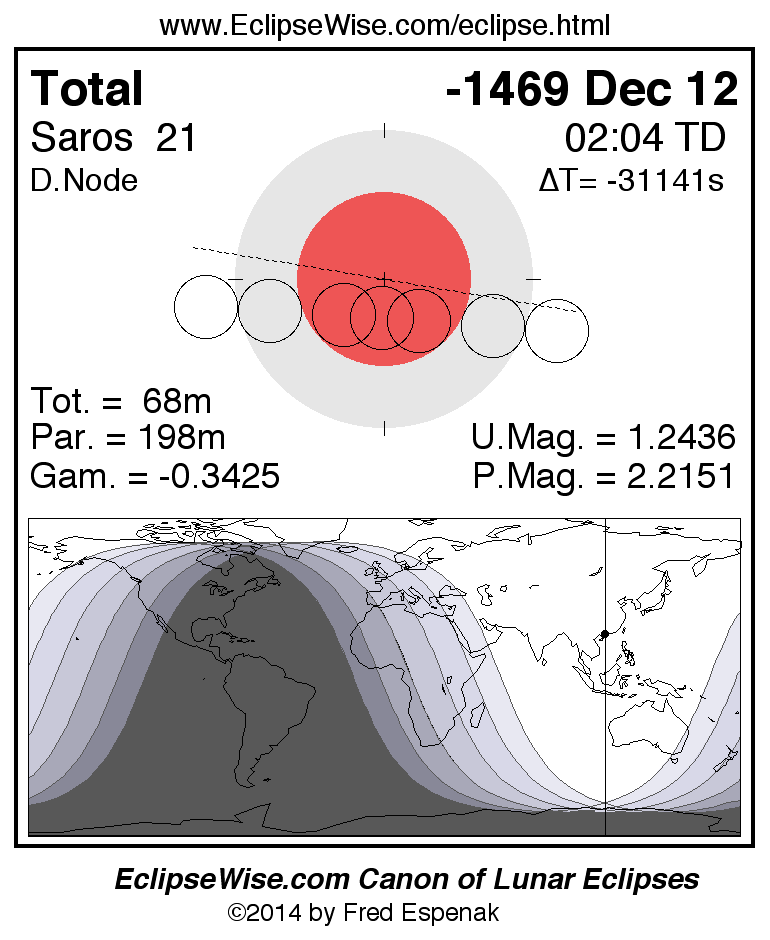
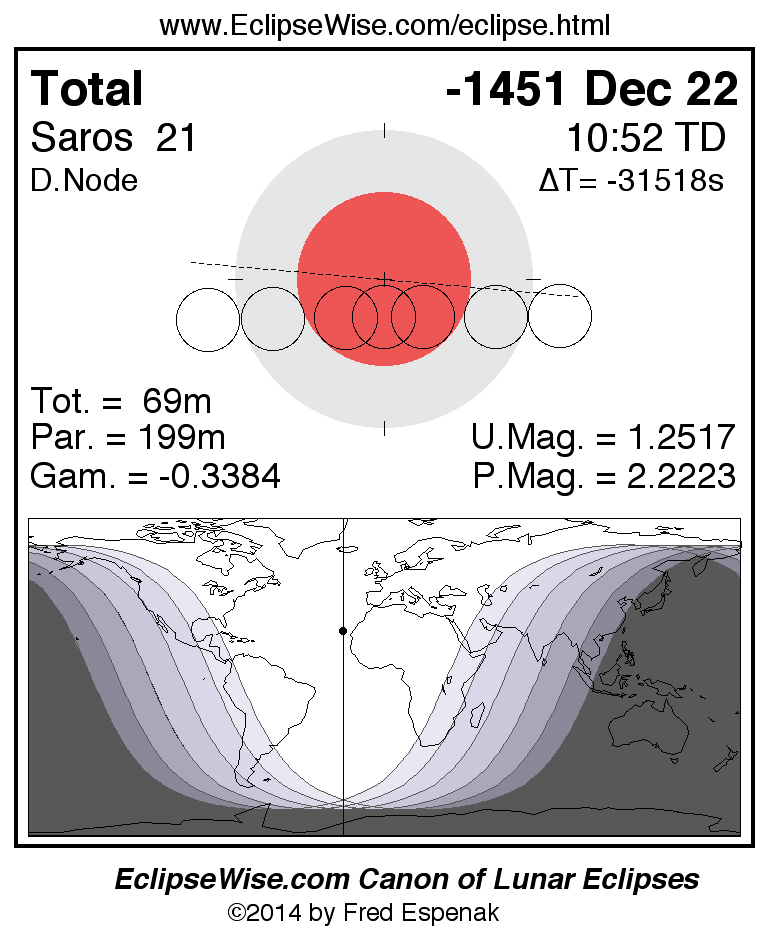
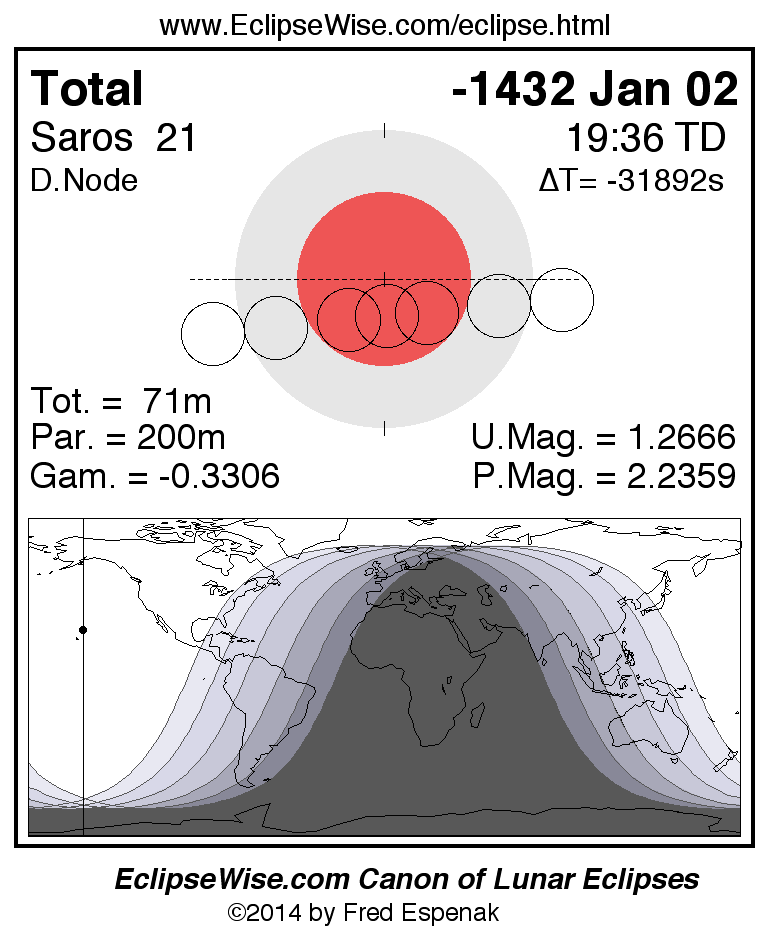
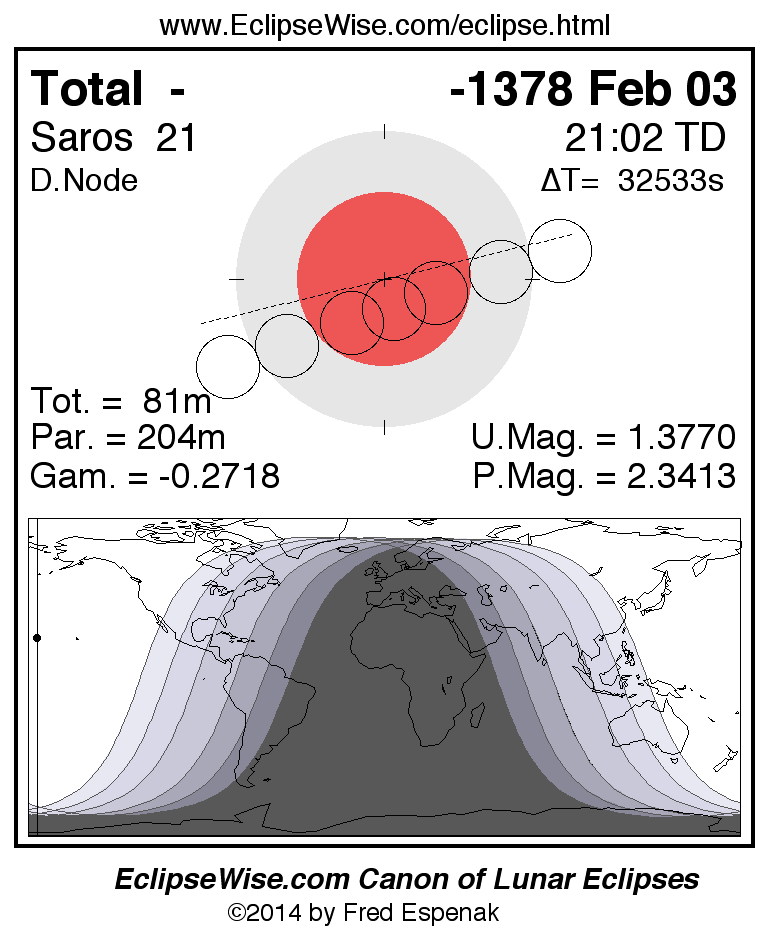
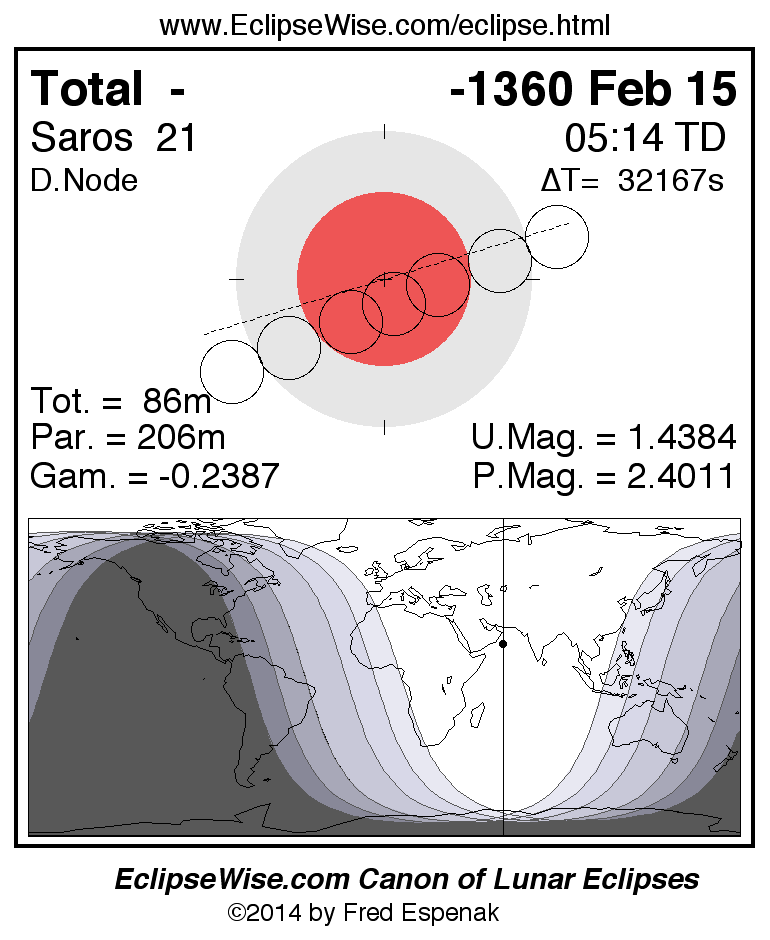
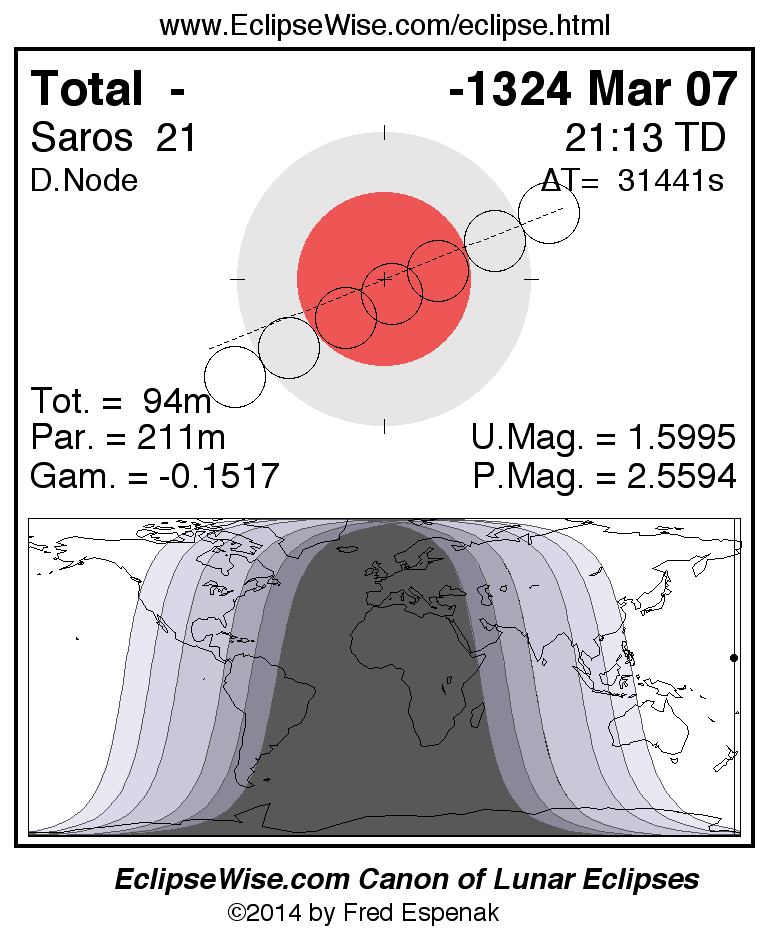
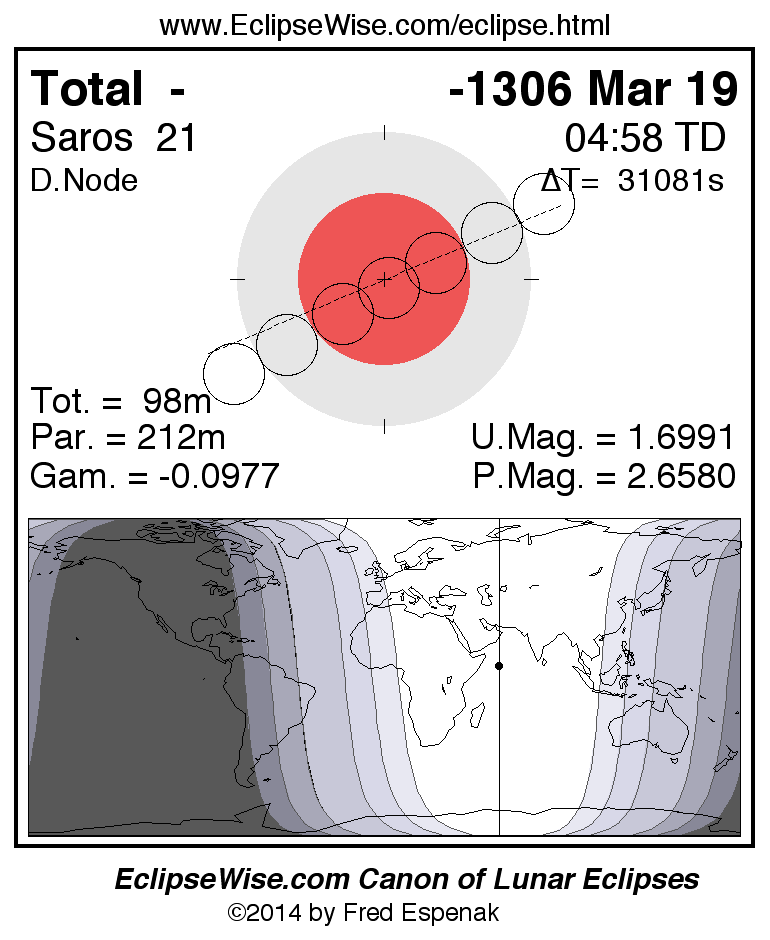
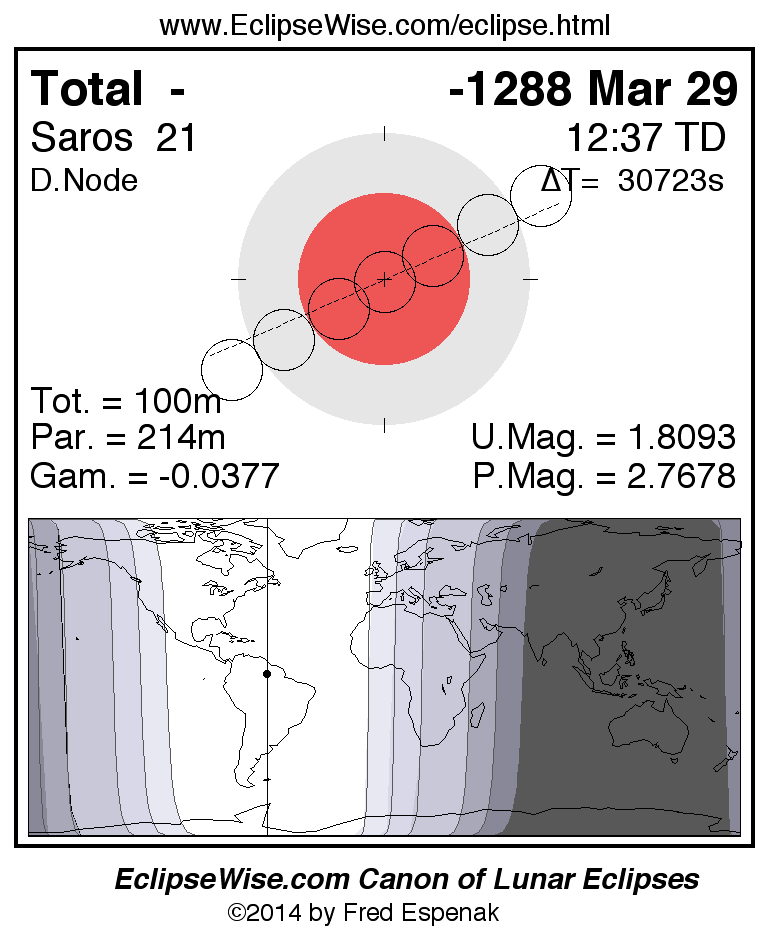
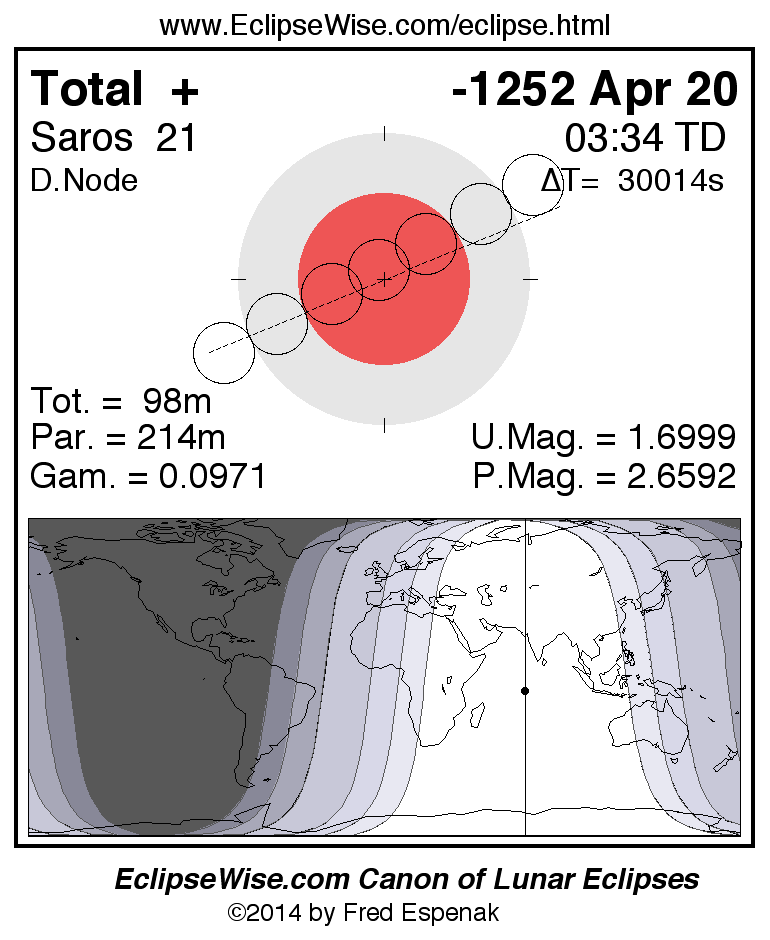
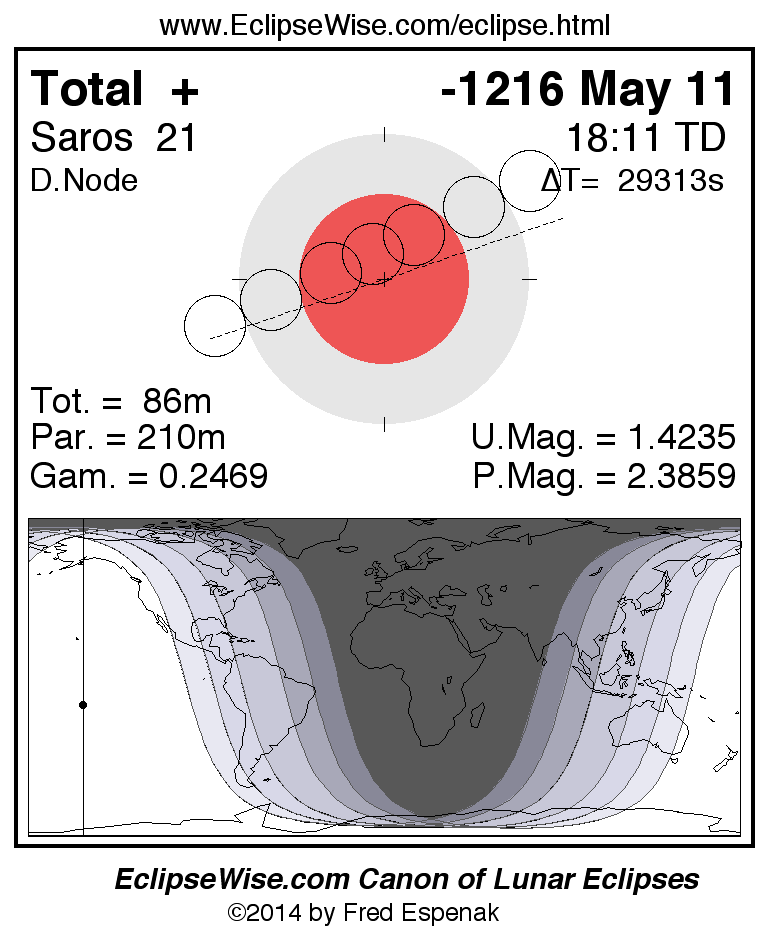
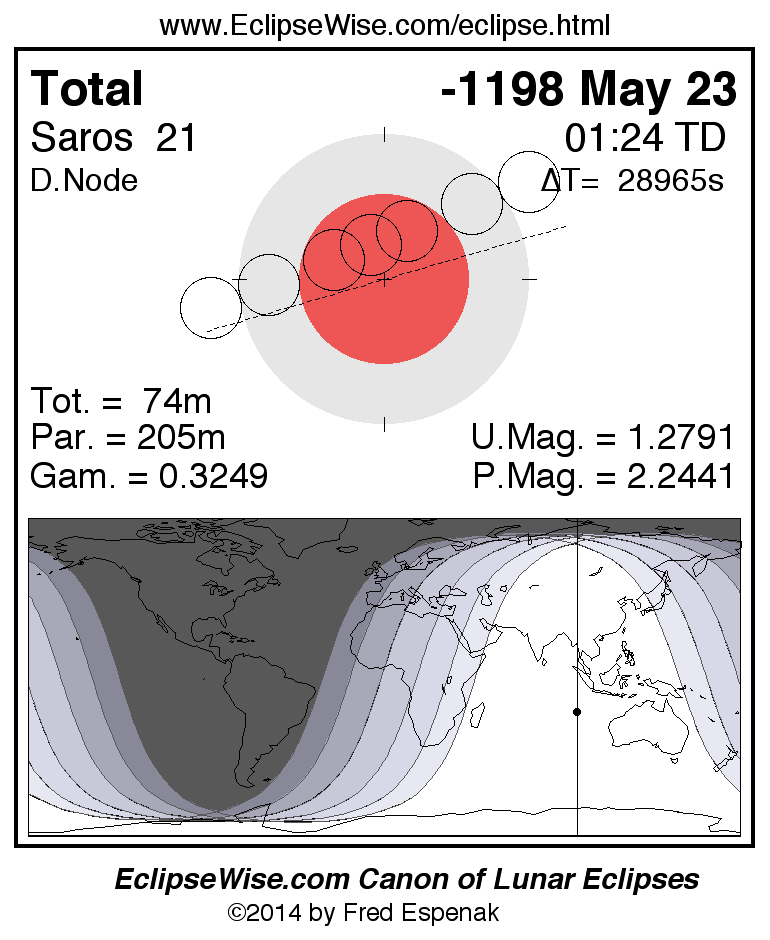
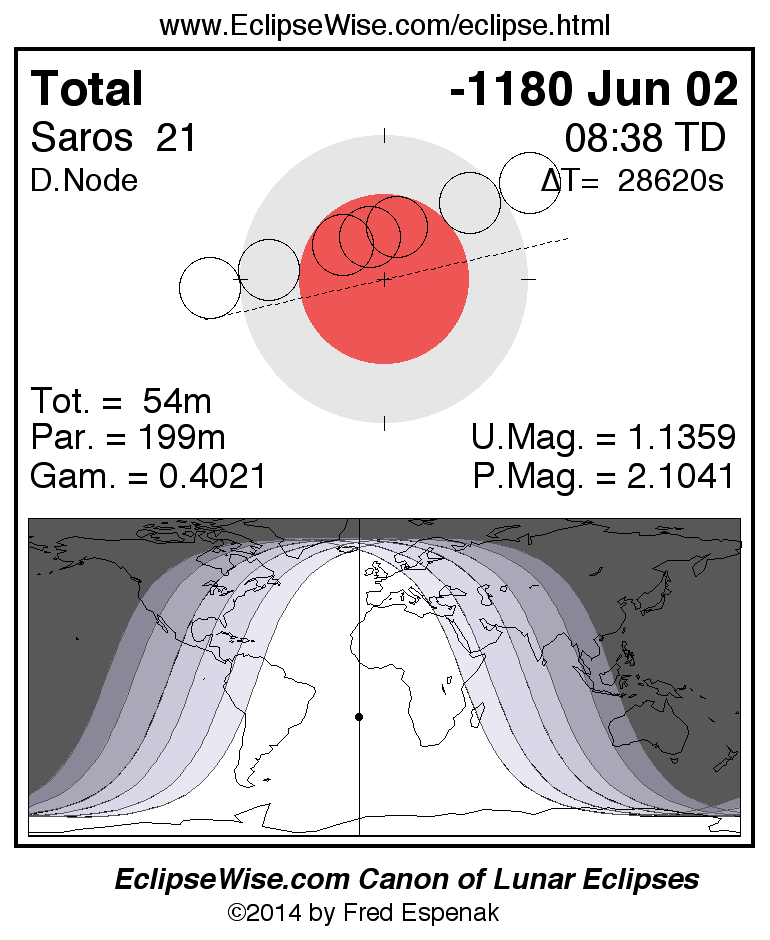
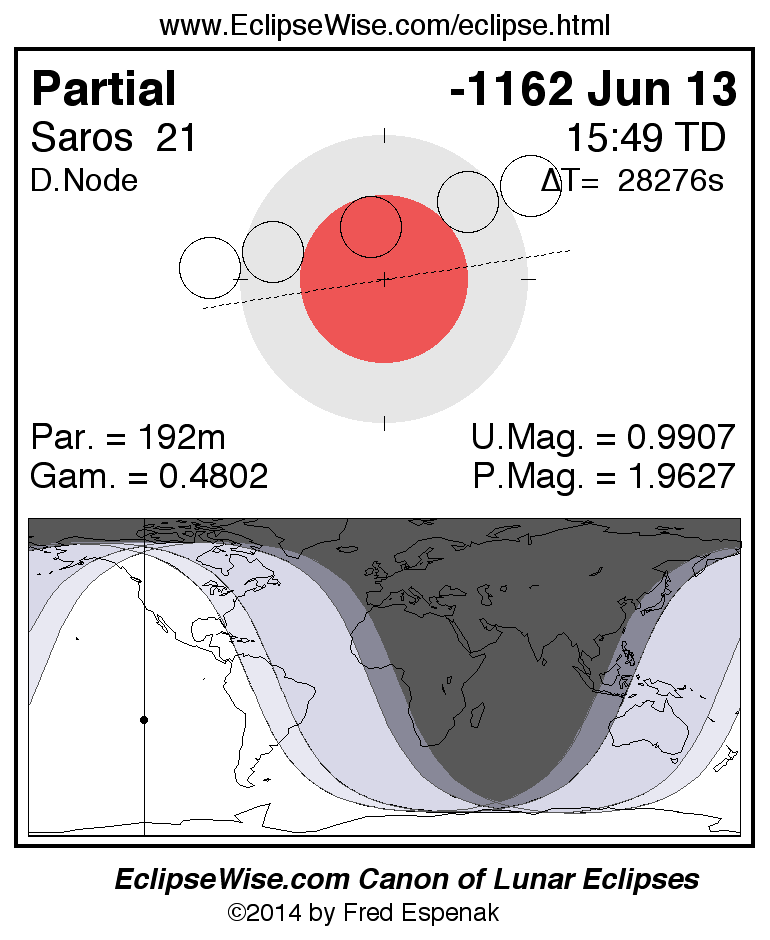
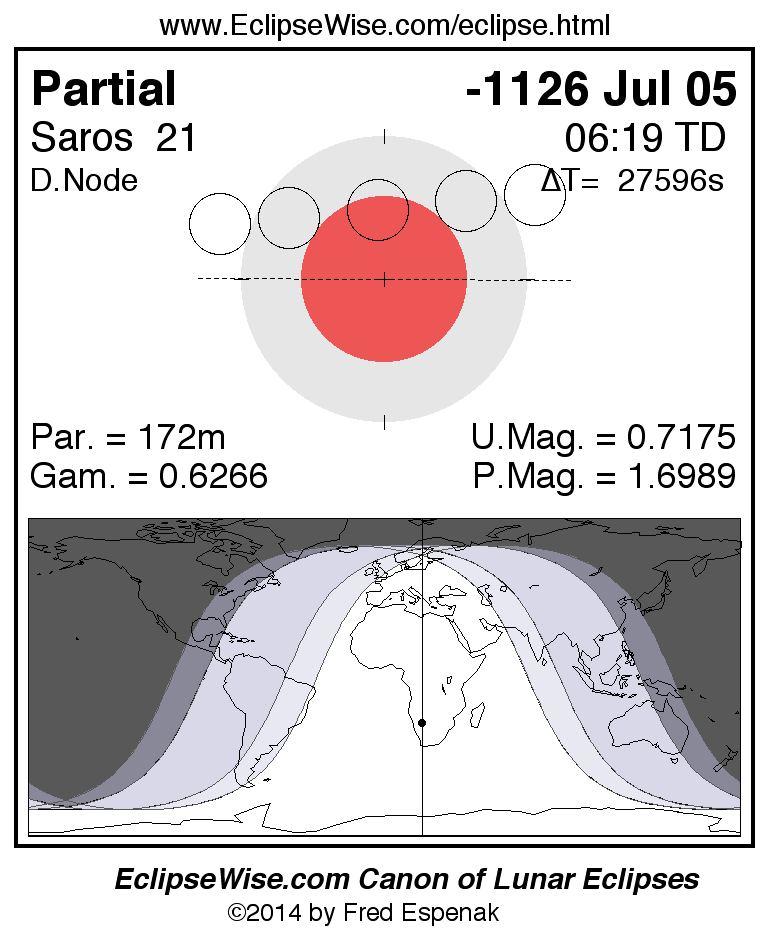
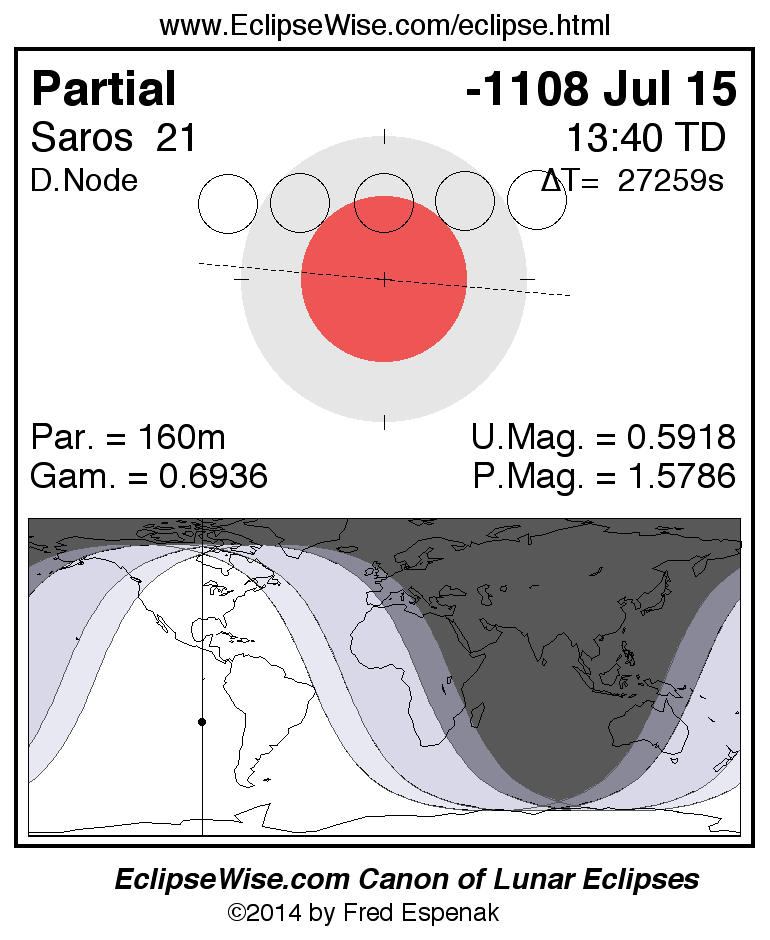
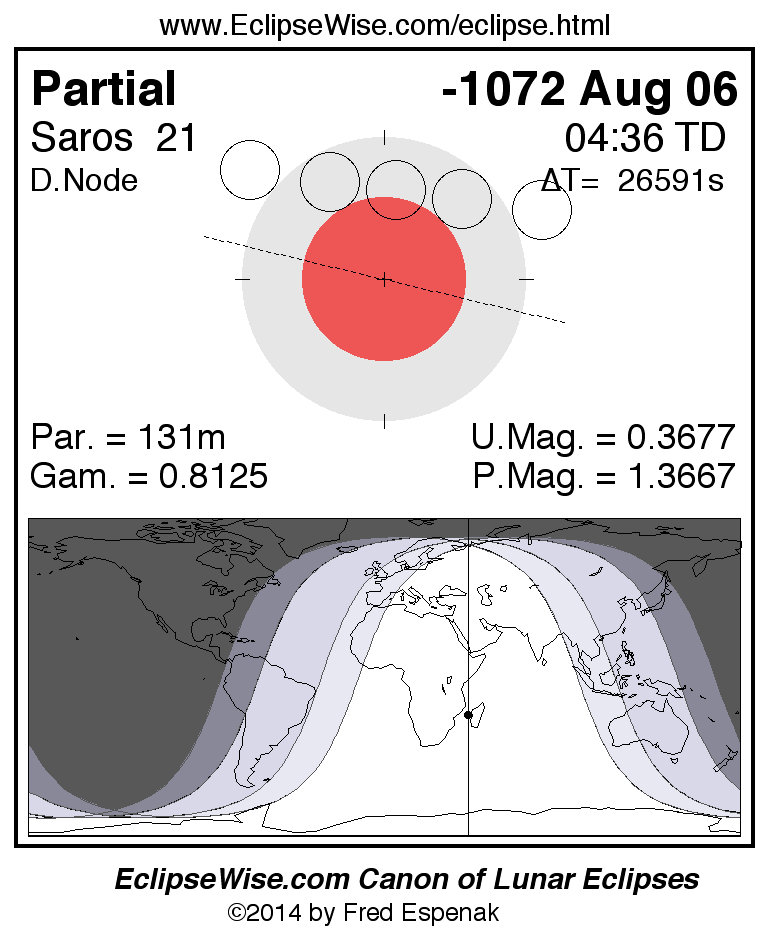
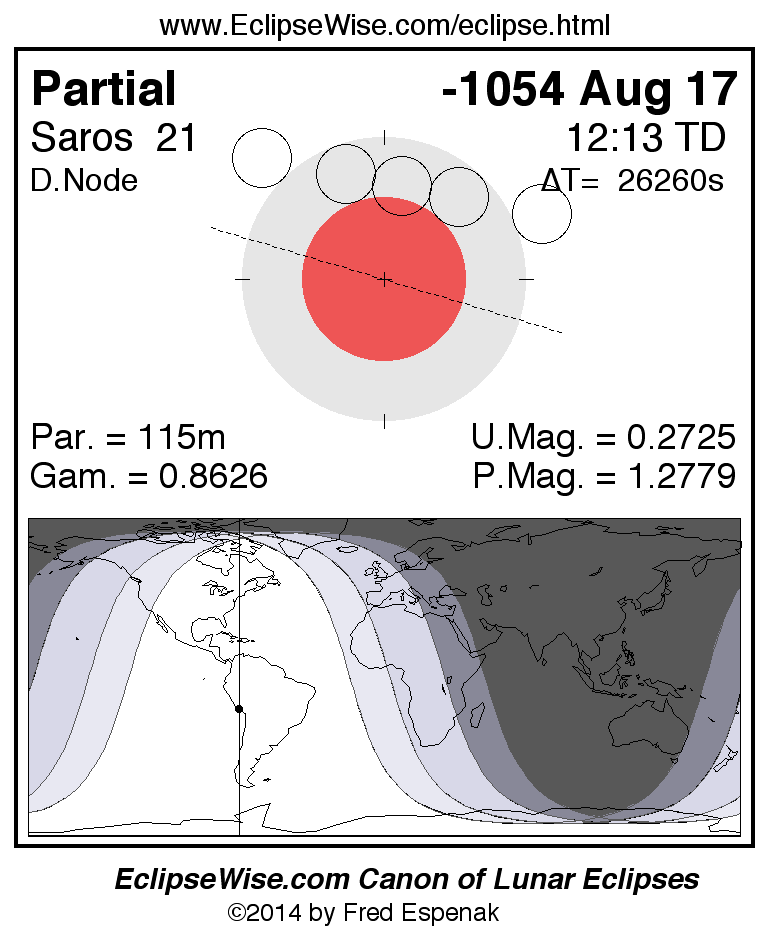
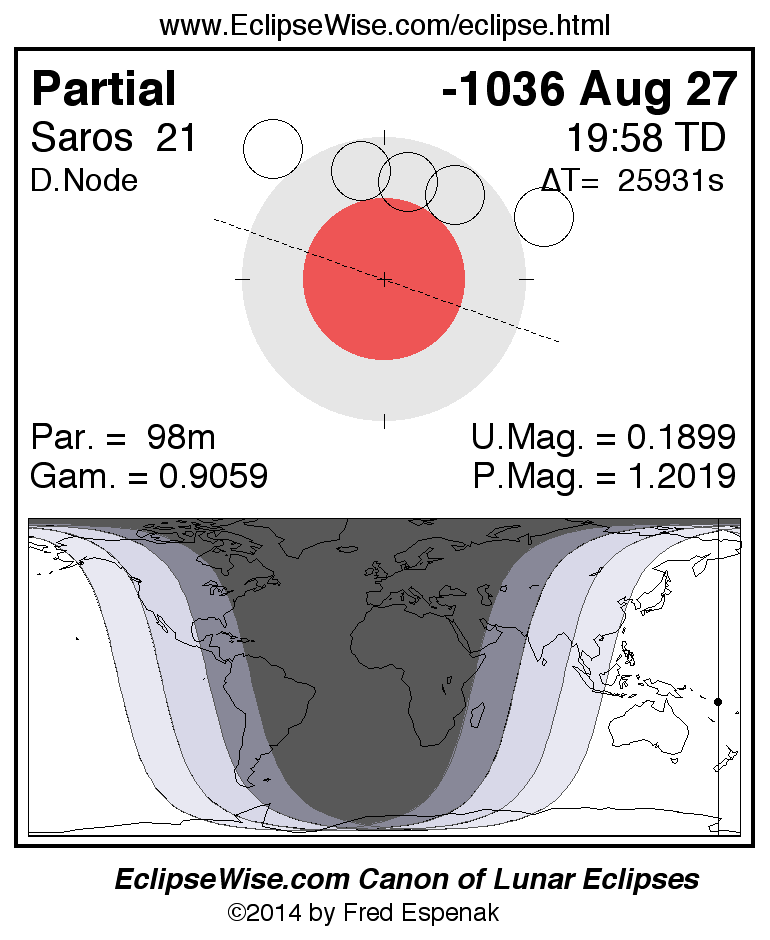
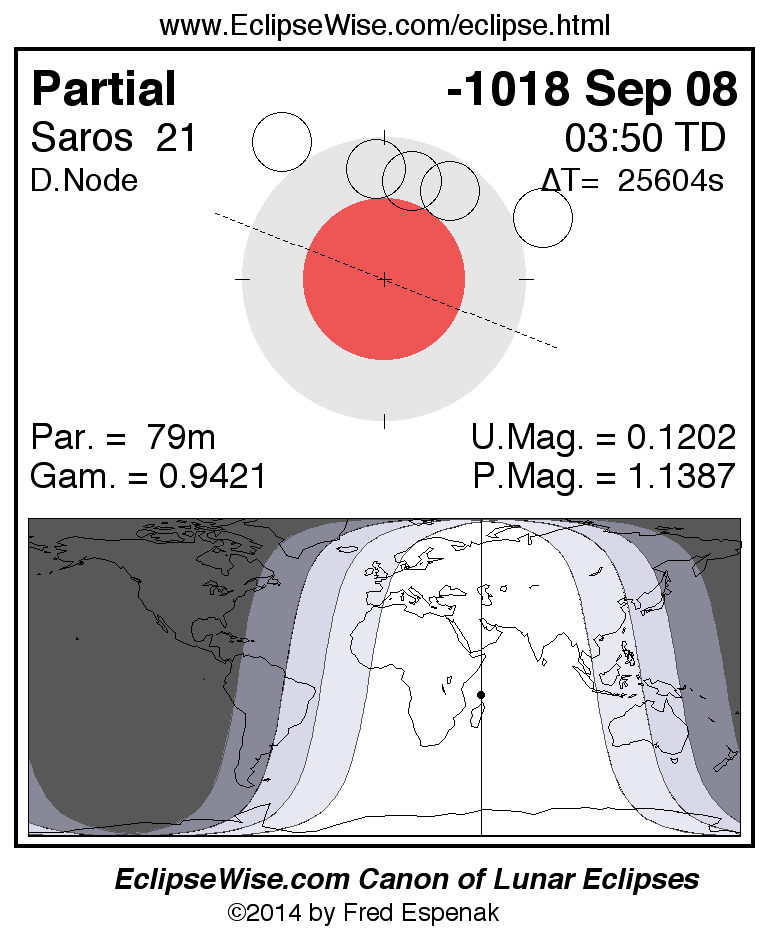
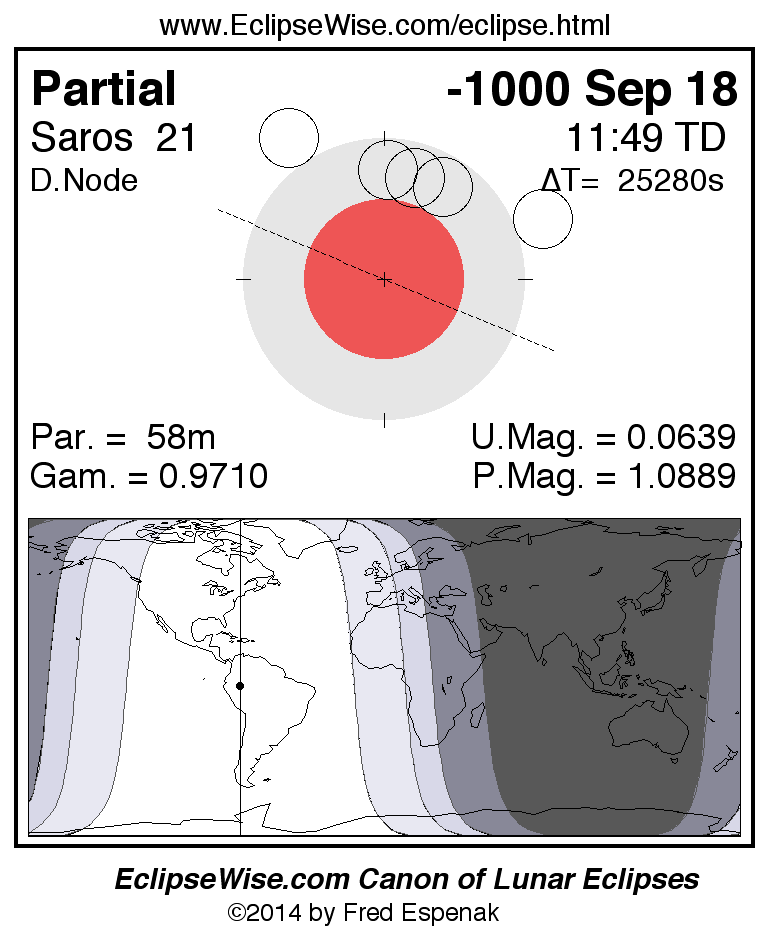
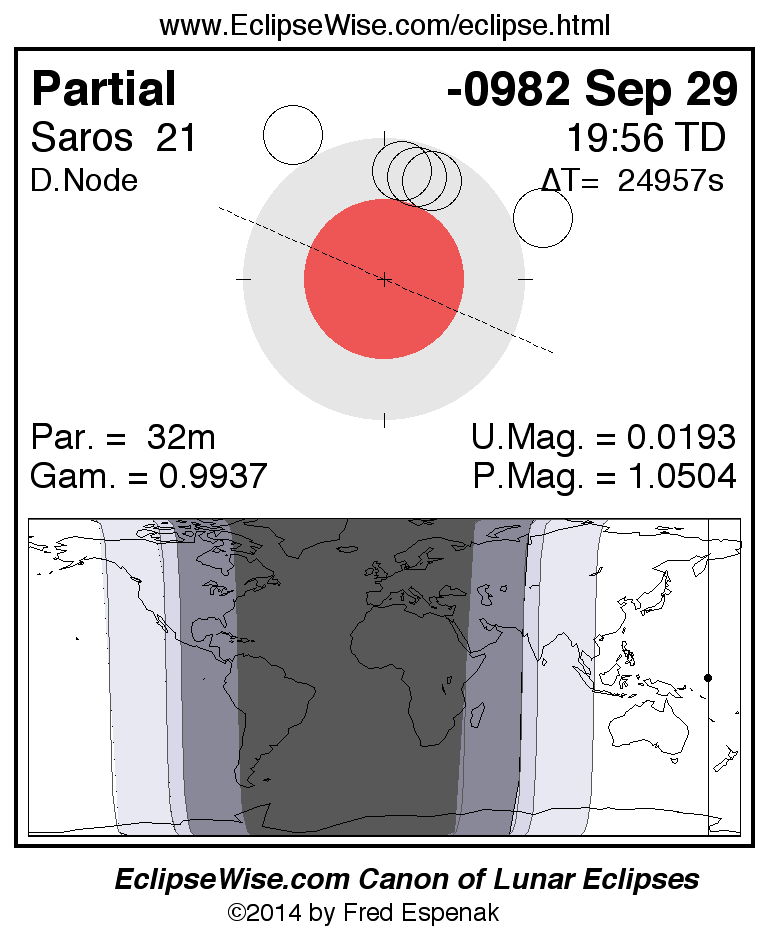
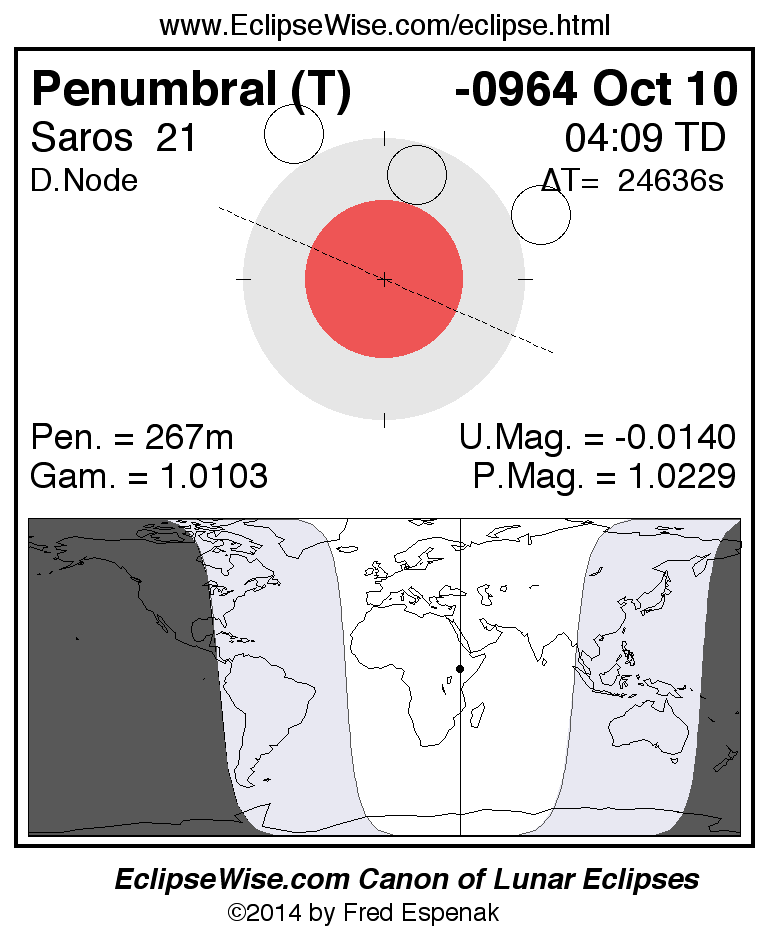
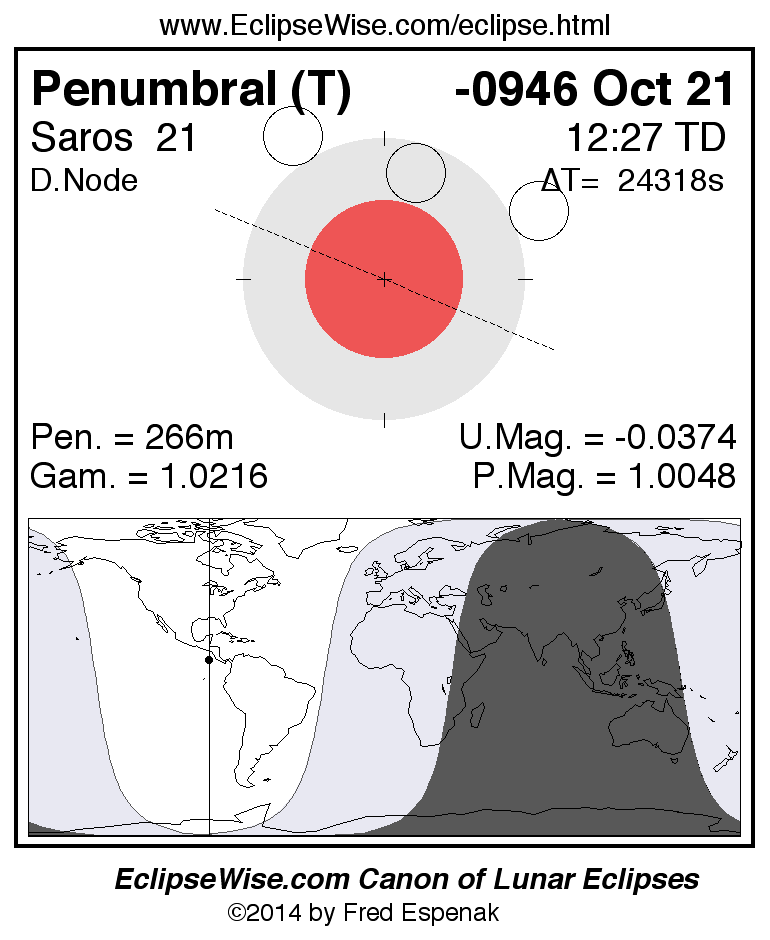
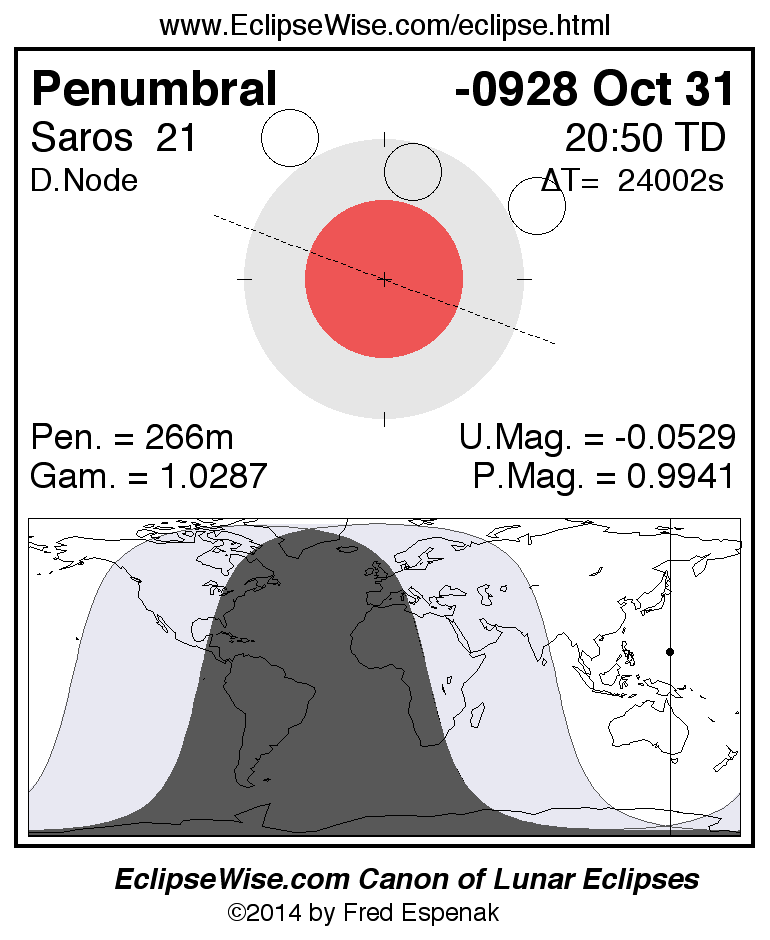
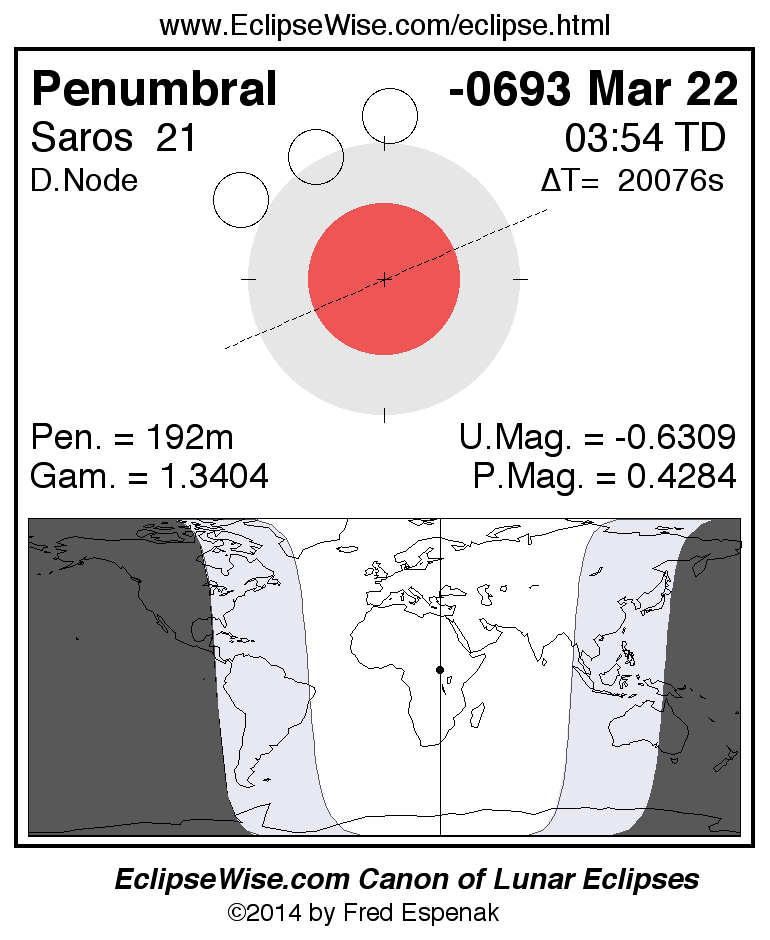
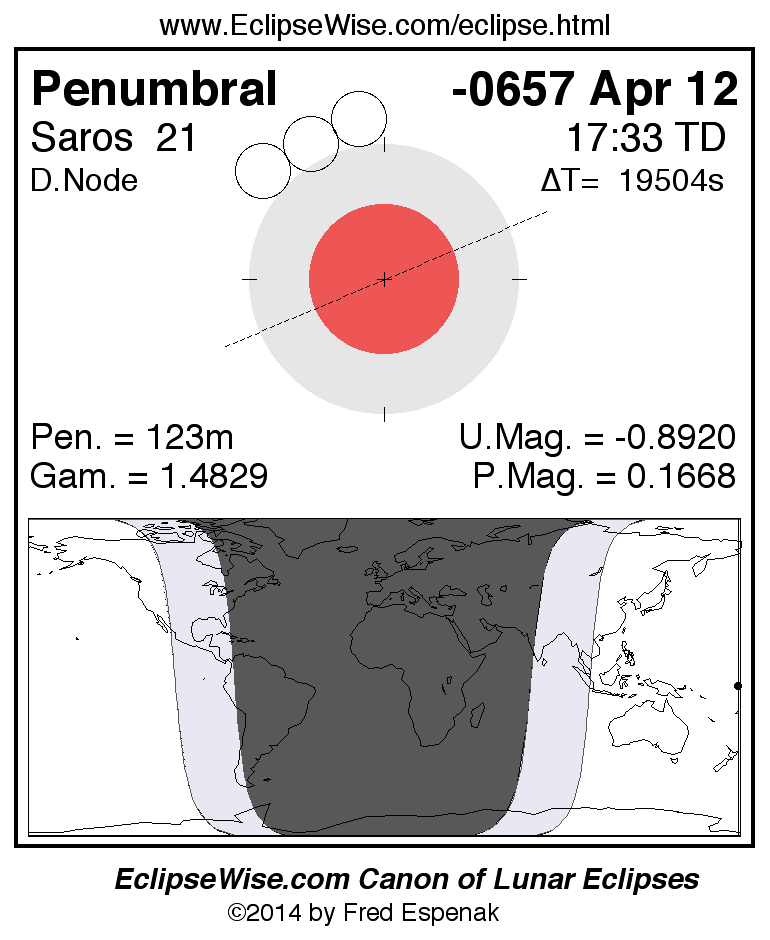
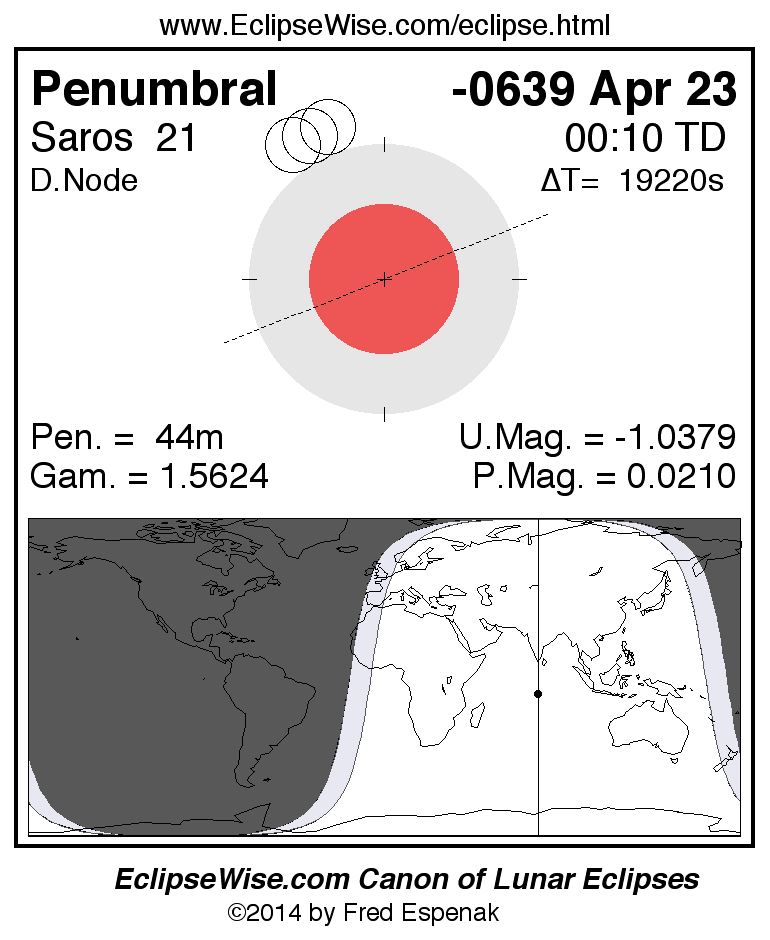
A panorama of all lunar eclipses belonging to Saros 21 is presented here. Each figure shows the Moon's path with respect to Earth's penumbral and umbral shadows. Below the path is a map depicting the geographic region of visibility for the eclipse. The date and time are given for the instant of Greatest Eclipse. Every figure serves as a hyperlink to the EclipseWise Prime page for that eclipse with a larger figure and complete details for the eclipse. Visit the Key to Lunar Eclipse Figures for a detailed explanation of these diagrams. Near the bottom of this page are a series of hyperlinks for more on lunar eclipses.
The exeligmos is a period of three Saros cycles and is equal to approximately 54 years 33 days. Because it is nearly an integral number of days in length, two eclipses separated by 1 exeligmos (= 3 Saroses) not only share all the characterists of a Saros, but also take place in approximately the same geographic location.
The Saros panorama below is arranged in horizontal rows of 3 eclipses. So one eclipse to the left or right is a difference of 1 Saros cycle, and one eclipse above or below is a difference of 1 exeligmos. By scanning a column of the table, it reveals how the geographic visibility of eclipses separated by an exeligmos slowly changes.
- Click on any figure to go directly to the EclipseWise Prime Page for more information, tables, diagrams and maps. Key to Lunar Eclipse Figures explains the features in these diagrams.
For more information on this series see Statistics for Lunar Eclipses of Saros 21 .
Statistics for Lunar Eclipses of Saros 21
Lunar eclipses of Saros 21 all occur at the Moon’s descending node and the Moon moves northward with each eclipse. The series will begin with a penumbral eclipse near the southern edge of the penumbra on -1955 Feb 22. The series will end with a penumbral eclipse near the northern edge of the penumbra on -0639 Apr 23. The total duration of Saros series 21 is 1316.20 years.
| Summary of Saros 21 | |
| First Eclipse | -1955 Feb 22 |
| Last Eclipse | -0639 Apr 23 |
| Series Duration | 1316.20 Years |
| No. of Eclipses | 74 |
| Sequence | 8N 8P 28T 11P 19N |
Saros 21 is composed of 74 lunar eclipses as follows:
| Lunar Eclipses of Saros 21 | |||
| Eclipse Type | Symbol | Number | Percent |
| All Eclipses | - | 74 | 100.0% |
| Penumbral | N | 27 | 36.5% |
| Partial | P | 19 | 25.7% |
| Total | T | 28 | 37.8% |
The 74 lunar eclipses of Saros 21 occur in the order of 8N 8P 28T 11P 19N which corresponds to the following.
| Sequence Order of Lunar Eclipses in Saros 21 | ||
| Eclipse Type | Symbol | Number |
| Penumbral | N | 8 |
| Partial | P | 8 |
| Total | T | 28 |
| Partial | P | 11 |
| Penumbral | N | 19 |
The 74 eclipses in Saros 21 occur in the following order : 8N 8P 28T 11P 19N
The longest and shortest eclipses of Saros 21 as well as largest and smallest partial eclipses appear below.
| Extreme Durations and Magnitudes of Lunar Eclipses of Saros 21 | |||
| Extrema Type | Date | Duration | Magnitude |
| Longest Total Lunar Eclipse | -1270 Apr 09 | 01h40m05s | - |
| Shortest Total Lunar Eclipse | -1667 Aug 14 | 00h18m08s | - |
| Longest Partial Lunar Eclipse | -1162 Jun 13 | 03h11m56s | - |
| Shortest Partial Lunar Eclipse | -0982 Sep 29 | 00h32m10s | - |
| Longest Penumbral Lunar Eclipse | -0856 Dec 14 | 04h27m41s | - |
| Shortest Penumbral Lunar Eclipse | -0639 Apr 23 | 00h44m27s | - |
| Largest Partial Lunar Eclipse | -1162 Jun 13 | - | 0.99070 |
| Smallest Partial Lunar Eclipse | -0982 Sep 29 | - | 0.01931 |
Links to Additional Lunar Eclipse Predictions
- Home - home page of EclipseWise with predictions for both Solar and lunar eclipses
- Lunar Eclipses - primary page for lunar eclipse predictions
- Lunar Eclipse Links - detailed directory of links
- Lunar Eclipse Basics - a primer on lunar eclipses
- Catalog of Lunar Eclipse Saros Series - covers Saros series -30 through 190
- Six Millennium Catalog of Lunar Eclipses - from -2999 to +3000 (3000 BCE to 3000 CE)
- Javascript Lunar Eclipse Explorer - calculate all lunar eclipses visible from a city
- Thousand Year Canon of Lunar Eclipses 1501 to 2500 - link to the publication
- Catalog of Lunar Eclipses of Historical Interest - includes a list of noteworthy lunar eclipses from history
- Lunar Eclipses of History (Reis) - a contributed article from Norma Reis
- MrEclipse.com - eclipse resources and tips on photography
- Lunar Eclipses for Beginners - a primer on lunar eclipse basics
- How to Photograph a Lunar Eclipse - instructions for imaging an eclipse of the Sun
- MrEclipse Photo Index - an index of lunar eclipse photographs
Calendar
The Gregorian calendar (also called the Western calendar) is internationally the most widely used civil calendar. It is named for Pope Gregory XIII, who introduced it in 1582. On this website, the Gregorian calendar is used for all calendar dates from 1582 Oct 15 onwards. Before that date, the Julian calendar is used. For more information on this topic, see Calendar Dates.
The Julian calendar does not include the year 0. Thus the year 1 BCE is followed by the year 1 CE (See: BCE/CE Dating Conventions). This is awkward for arithmetic calculations. Years in this catalog are numbered astronomically and include the year 0. Historians should note there is a difference of one year between astronomical dates and BCE dates. Thus, the astronomical year 0 corresponds to 1 BCE, and astronomical year -1 corresponds to 2 BCE, etc..
Eclipse Predictions
The eclipse predictions presented here were generated using the JPL DE406 solar and lunar ephemerides. The lunar coordinates have been calculated with respect to the Moon's Center of Mass.
The largest uncertainty in the eclipse predictions is caused by fluctuations in Earth's rotation due primarily to tidal friction of the Moon. The resultant drift in apparent clock time is expressed as ΔT and is determined as follows:
- pre-1950's: ΔT calculated from empirical fits to historical records derived by Morrison and Stephenson (2004)
- 1955-present: ΔT obtained from published observations
- future: ΔT is extrapolated from current values weighted by the long term trend from tidal effects
A series of polynomial expressions have been derived to simplify the evaluation of ΔT for any time from -2999 to +3000. The uncertainty in ΔT over this period can be estimated from scatter in the measurements.
Acknowledgments
Some of the content on this web site is based on the books Five Millennium Canon of Lunar Eclipses: -1999 to +3000 and Thousand Year Canon of Lunar Eclipses 1501 to 2500. All eclipse calculations are by Fred Espenak, and he assumes full responsibility for their accuracy.
Permission is granted to reproduce eclipse data when accompanied by a link to this page and an acknowledgment:
"Eclipse Predictions by Fred Espenak, www.EclipseWise.com"
The use of diagrams and maps is permitted provided that they are NOT altered (except for re-sizing) and the embedded credit line is NOT removed or covered.